thx but i wanted derivation of (1) only
Q1. Light of intensity I falls on a perfectly reflecting surface at an angle θ with the normal to the surface. Find the force exerted by the light on the surface.
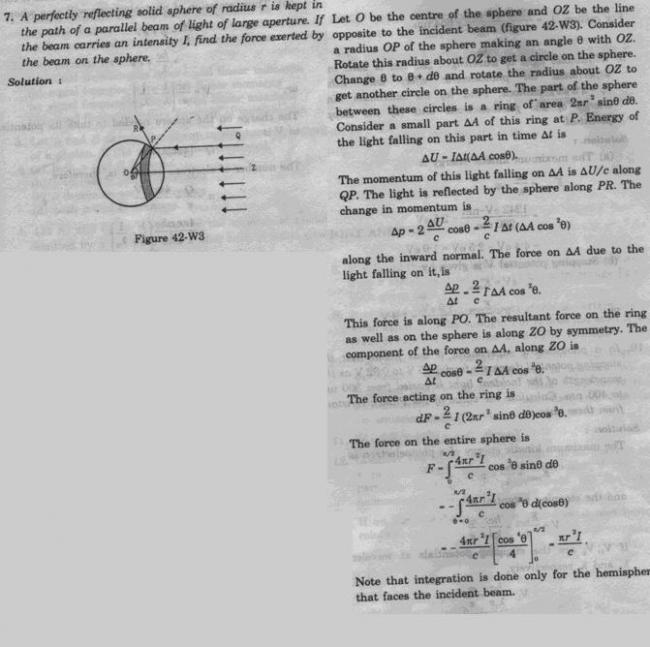
Q2. A perfectly reflecting solid sphere of radius r is kept in the path of a parallel beam of light of large aperture. If the beam carries an intensity I, find the force exerted by the beam on the sphere
-
UP 0 DOWN 0 0 7

7 Answers
can u pls explain that..
i really dont want the ans to second one ..
i just req derivation of first one
2)
Incident raditaion at diff angle will prdouce diff radiation presure
Take an leementry ring radius r
P=2Icos2θc
dF=P.ds
Perpendicular components cancel out
So dF cosθ=Pcosθds
=> Fnet=∫ Pcosθds
now just put the values
okk
i will derive for simple normal incidence...u try for incidence at angle...its easy
Momentum eqns ::
pi=nh/λ
Since elastic surface,so,
pf=nh/λ
Impulse eqn:
-J=pf-(-pi)
J=2nh/λ
F=2nh/λt
Pressure=2nh/λSt S=Area
Now just manipulate it..
Pressure=2nhc/λStc
we know ,energy=nhc/λ
power=nhc/λt
Intensity=nhc/λtS
=>Pressure=2(nhc/λStc)=2I/c
still in need of answer (derivation) for first part.. (i think it is 2Icosθ/c)
btw
about the second question, this is HCV solution .. can anyone explain it step-by-step please ?? Help this poor kid please.. (ive gotten tired now requesting)
Derivation & Shortcut for solving these type of questions:
We know Intensity (I) = Energy/Area*Time
or, I = F.dsArea*dt
or, I = F*cA (Since, ds/dt for a photon is c, the velocity of light)
or, F = I*Ac
Now for calculating the force on the sphere in the path of the beam of light, it is similar to calculating the force experienced by the Earth due to the Sun's Rays (one part of the Earth is lighted and the other part is dark).
Now, a person standing at the Equator on a surface area of A will experience F = I*Ac
But, for a person at the poles, it will be zero (Intensity is calculated by taking the area as always perpendicular to the direction of the rays)
So, for a person standing between the Equator and the poles, say at an angle θ, the force will be, F = I*Acosθc, Now summing the area up is nothing but the projection of a hemisphere (flattening a hemisphere with a rod), which is a circle of same radius.
Hence answer comes out as: F = I*Λr2c where r is the radius of the sphere