olympiad math

We prove that whenever \sum_{i=1}^N{x_i^2} \ge ab+(a+b)x_1,....(1)
squares of sides x_1\ge x_2 \ge x_3 \ge \cdots \ge x_N will cover a rectangle of side aXb.
For N=1, claim is trivial.
Let for N=1,2,...,m-1, the claim is true.
Then now let N=m and j be least integer such thatx_1+ x_2 +x_3 + \cdots + x_j \ge a
We cover the part a\times x_j with squares of side length x_1,...,x_j.
The remaining can be covered by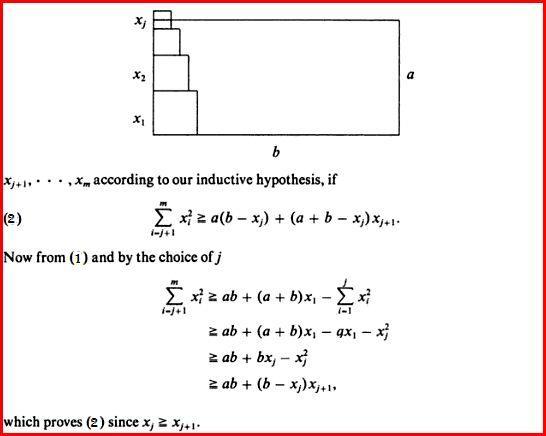

So induction is complete and our claim is proved.
in our case a=b=1.
and the sigma is 3.

wah wat a sol ith power !!!
b555 asked for a chocolate and u ve given him a cake !!!

did u come across this q previously ???
Im not able to imagine how u cud have imagined to prove it for all rectangles wen q is askin for square alone that too with narrow values

may i know the name of the book ith power? is it a ebook or a real one?

k i thought u were an alien after seeing #3 :)

btw i am not even able to understand that exp
thinking hard