74. A particle is moving at a constant speed V from a large distance towards a concave mirror of radius \R along its principal axis. Find the speed of the image formed by the mirror as a function of distance x of the particle from the mirror.
Q73 A ball is kept at a height of h above the surface of a heavy transparent sphere made of a material of refractive index μ. The radius of the sphere is R. At t=0, the ball is dropped to fall normally on the sphere. Find the speed of the image formed as a function of time for t<√2h/g. Consider only the image by a single refraction
-
UP 0 DOWN 0 0 13

13 Answers
75 A small block of mass m and a concave mirror of radius R fitted with a stand lie on a smooth horizontal table with a separation d between them. The mirror together with its stand has a mass m. The block is pushed at t=0 towards the mirror so that it starts moving towards the mirror at a constant speed V and collides with it. The collision is perfectly elastic. find the velocity of the image (a) at a time t<d/V
(b) at a time t>d/V
76 A gun of mass M fires a bullet of mass m with a horizontal speed V. The gun is fitted with a concave mirror of focal length f facing towards the receding bullet. Find the speed of separation of the bullet and the image just after the gun was fired.
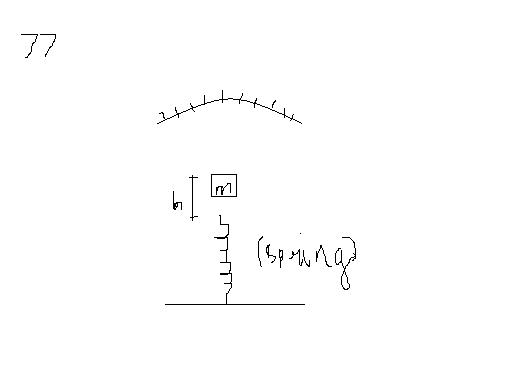
77 A mass m=50g is dropped on a vertical spring of spring constant 500N/m from a height h=10cm as shown in figure. The mass sticks to the spring executes simple harmonic oscillations after that. A concave mirror of focal length 12cm facing the mass is fixed with its principal axis coinciding with the line of motion of the mass, its pole being at a distance of 30cm from the free end of the spring. Find the length in which the image of the mass oscillates.
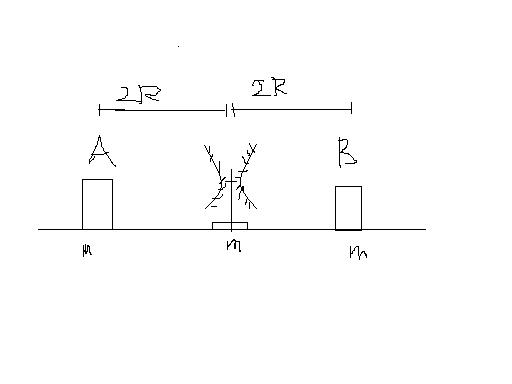
78 Two concave mirrors of equal radii of curvature R are fixed on a stand facing opposite directions. The whole system has a mass m and is kept on a frictionless horizontal table.
Two blocks A and B each of mass m are placed on the two sides of the stand. At t=0, the separation between A and the mirrors is 2R. The block B moves towards the mirror at a speed v. All collisions which take place are elastic. Taking the original position of the mirrost stand system to be x=0 and X axis along AB find the positions of the images of A and B at t=
a)R/v
b)3R/v
c)5R/v
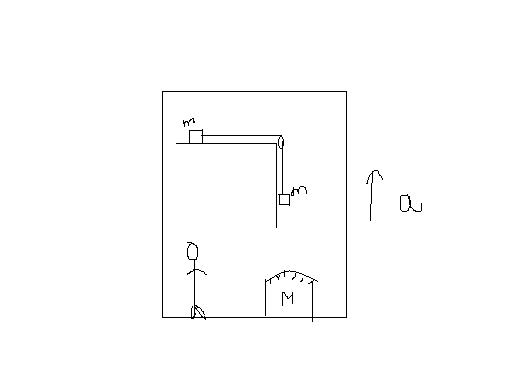
79. Consider the situation show in figure. The elevator is going up with an acceleration of 2.00 m/s2 and the focal length of the mirror is 12 cm. All the surfaces are smooth and the pulley is light. The mass-pulley is released from rest(with respect to the elevator) at t=0 when the distance of B from the mirror is 42.0cm. Find the distance between the image of the block B and the mirror at t=0.200s. Take g=10m/s2
thanx subash for ur effort..........now i will do these questions.......[1][1][1]