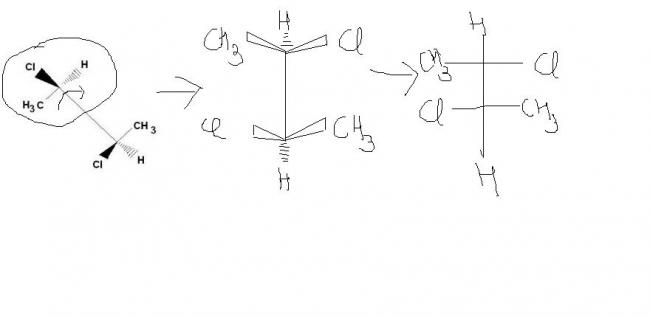
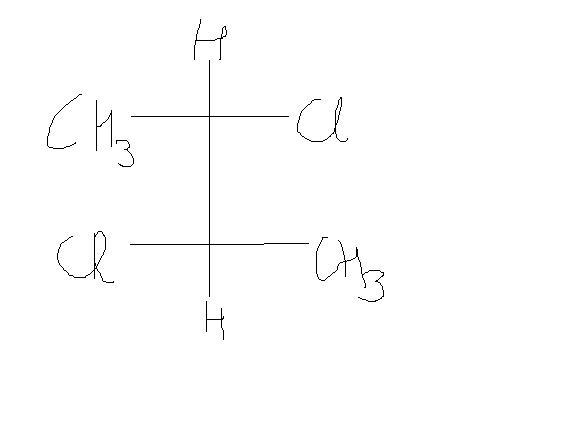
11one ch3 on the other side
The correct statement(s) about the compound given below is (are)
(A) The compound is optically active
(B) The compound possesses centre of symmetry
(C) The compound possesses plane of symmetry
(D) The compound possesses axis of symmetry
-
UP 0 DOWN 0 1 26
26 Answers
1in place of doing so much work whhy couldn't we go for r/s notation ,which will readily say whether its opticallty active or not..
if found optically in active now look for various symmetry.
e.g. its R for both carbons threrefore its opt. active
simple
one more thing do anyone know whether optically active compounds posses any
(B) The compound possesses centre of symmetry
(C) The compound possesses plane of symmetry
(D) The compound possesses axis of symmetry
33yes.. thats true but this was not about the answer but the method convert 3d to fischer and to see the elements of symmetry... [1]
1@@@@@@@@@@@@@@@@@@@@@@@@@@@@@@@@
wenevr a molecule is optically active... jus forget
>> plane of symmatry
>> centre of symmetry
>> 'alternate' axis of symmetry
but..... there CAN BE 'AXIS OF SYMMETRY'...
@@@@@@@@@@@@@@@@@@@@@@@@@@@@@@@@
1thanksssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssssss
33
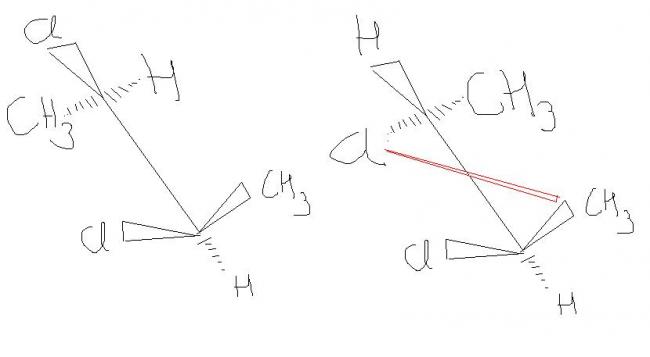
first make any atom on both carbons opposite the point 'P'(central point..)( in this case i have made -H) by rotating at any one carbon..
now u can see (red line is coming out of paper.. from Cl to CH3..passing through P) that on moving from P one side you will get Cl other side CH3
1thankssssssssssss............... but priyam .... kya centre mein point of symmetry hoga ?????????? becoz moving in opposite direction we get same molecule...............
33just by rotating groups at both carbons so that... two are out of plane and one behind plane in between...(make this at both carbons..) and thats ur fischer projection....
33yaar mere ko fischer nahi thik se dikhta hai... 3d is better..
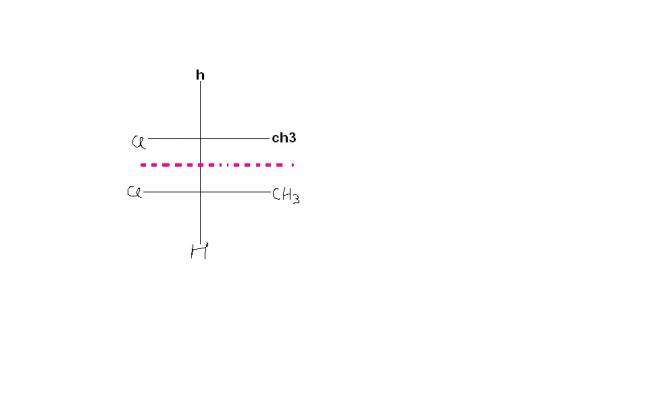
33no.. plane of symmetry and point of symm is not there... just see once more..
1and also ,,,, plane bisecting CH3 or plane parallel to the plane of page will be a plane of symmetry.
correct me if m wrong
33draw its mirror image and you will notice that they are not super imposable.. thats it..