1
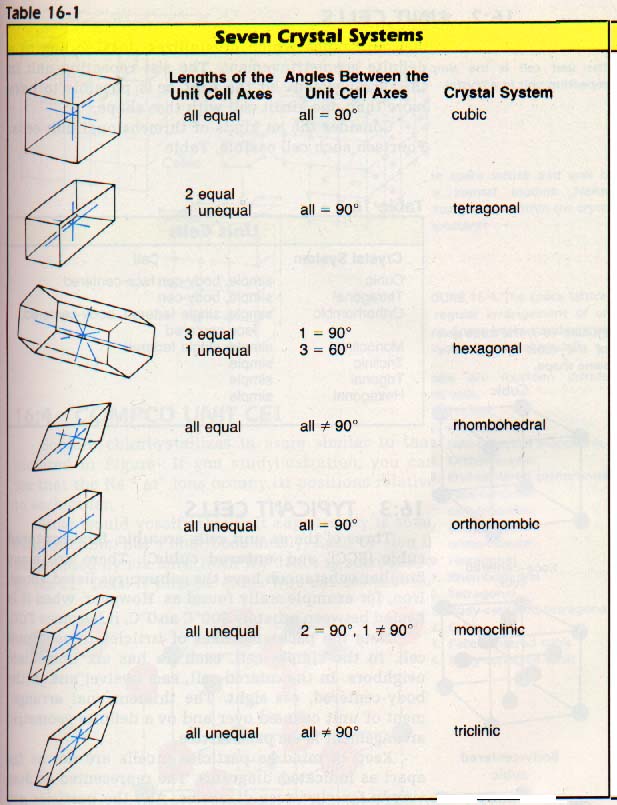
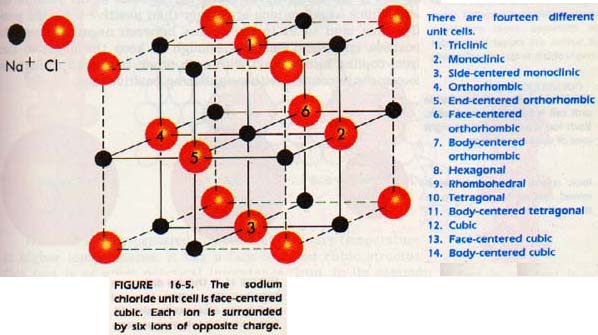
cofumation wanted.........
In case of a cubic cell havin atoms in CCP latticve, at EDGE-CENTRES at FACE CENTERE and at BODY - CENTER
relation b/w r of atom and edge length is : ??
is it 4r = a ??
and wats the ratio of radius of voids formd to radius of atoms
-
UP 0 DOWN 0 3 20
20 Answers
1
space used
= V(particles) /V(crystal cell )
= (4/3)Ï€(a/2)3 / a3
= 52.36%
Coordination number: 8
space used
= V (particles) / V (crystal cell )
= [2×(4/3)πr3 ]/a3
= 2×4/3πr3
= 68.02%
Coordination number: 12
space used
= V (particles) /V (crystal cell )
= 2×(4/3)π(a/2)3 /(a3×1.633a×sin1200 )
= 74.05%
1TETRAHEDRAL HOLE
OCTAHEDRAL HOLE
SIMPLE CUBIC
CN: 8:8
Cl- simple cubic
Cs+ simple cubic hole
Particles in one crystal cell
Cs+:1
CN: 4:4 S2- face-centered cubic
Zn2+ ½ tetrahedral hole
Particles in one crystal cell
Zn2+:4
1
Chalcopyrite has a very similar structure to sphalerite. Cells with iron atoms (orange) alternate with cells containing copper (blue). This double cell means chalcopyrite is tetragonal, but the fact that the unit cell consists of two cubes means chalcopyrite mimics cubic forms very often. In particular it can occur as pseudo-tetrahedra (actually tetragonal disphenoids, but they look exactly like tetrahedra.)
In sphalerite, we have a face-centered cubic arrangement of sulfur (yellow) and zinc in the center of each tetrahedron (purple). At left is the structure illustrating the relationship to the tetrahedra. At right is the unit cell. Zinc atoms hidden behind sulfur atoms are shown in a lighter shade.
Is this really ZnS? We have four zinc atoms entirely within the unit cell. We have eight corner sulfur atoms, but they are shared among eight unit cells. There are six sulfur atoms in the centers of the faces, but they are each shared with a neighboring cell. So we have 8 x 1/8 + 6 x 1/2 = 4 sulfur atoms. There are an equal number of zincs and sulfur.
1please can u mail all the relevant picture of solid state to me at my address ankitgoyalforyou@gmail.com
please reply
21thnx 4 da effrts!!!
but WER are EDGE CENTERed ATOMS???////
this is FCC....
1357yes it is 4r=a
If you see clearly, this is nothing but combination of 8 simple cubic unit cells of edge length a/2
after that it becomes very easy
1
in this type of unit cell the sphere at the center of the face is touching the end spheres
the radius of a sphere is r and the edge length is a
so the diagonal will be √2a
4r=√2a
=>a=2√2r
21WELL its not exactly wat i m askin.......
in ma Questn, Edge,Face n body all three are occupied.......
pl. givve the ans directly if posibl
1http://targetiit.com/iit_jee_forum/posts/18th_november_2008_583.html