Question Of the Day

24

eureka123 ·2009-09-08 23:49:21

is it easy ?????

try it alll

UP 0 DOWN 0
Post on FacebookPost anonymously?

24

eureka123 ·2009-09-13 11:12:59

no one trying ??

UP 0 DOWN 0
Post on FacebookPost anonymously?

1

injun joe ·2009-09-14 22:28:29

Let f₁= ax+b and f₂= cx+d
As f₁ and f₂ are onto,

-a+b=0
a+b=k
-c+d=k
c+d=0

Solving, we get f₁ and f₂ as
y=k/2(x) + k/2 = f₁ and
y=-k/2(x) + k/2 = f₂

From graph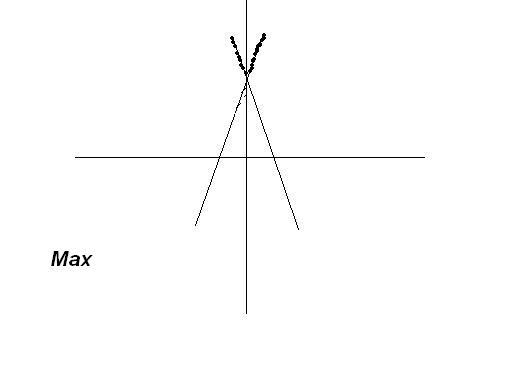

UP 0 DOWN 0
Post on FacebookPost anonymously?

1

injun joe ·2009-09-14 22:29:08

the point of intersection is (0,5k)

UP 0 DOWN 0
Post on FacebookPost anonymously?

24

eureka123 ·2009-09-14 22:32:01

what is c and d u have taken ??

also k has to be eliminated ..

so ur ans is not rite..

UP 0 DOWN 0
Post on FacebookPost anonymously?

1

injun joe ·2009-09-14 22:35:15

c and d are arbitrary constants..
And about k, .... :(

UP 0 DOWN 0
Post on FacebookPost anonymously?

24

eureka123 ·2009-09-14 22:41:16

and hint:
dont assume f₁x) and f₂(x) separately..instead generate them from a single equation f(x) using the conditions

UP 0 DOWN 0
Post on FacebookPost anonymously?

24

eureka123 ·2009-09-15 10:37:38

OK..seems like some one is tryign the ques...

so i will drop a hint

Let f(x)=ax+b
Now use the condition of onto mapping from [-1,1] to [0,k]
and find values of a and b in terms of k

UP 0 DOWN 0
Post on FacebookPost anonymously?

3

msp ·2009-09-16 20:10:58

can we assume f₁(x) and f₂(x) as y=x and y=-x respectively.

UP 0 DOWN 0
Post on FacebookPost anonymously?

24

eureka123 ·2009-09-16 20:37:46

No

UP 0 DOWN 0
Post on FacebookPost anonymously?

24

eureka123 ·2009-10-03 11:19:44

Looks like everyone has given up on this

UP 0 DOWN 0
Post on FacebookPost anonymously?

1

Arshad ~Died~ ·2009-10-03 11:21:57

ya dude......tried to some extent........but couldnt get it

UP 0 DOWN 0
Post on FacebookPost anonymously?

03-09-09

12 Answers

Your Answer

Similar Questions

03-09-09

12 Answers

Your Answer

Add Image

Similar Questions