11a , b,c are in Ap or Gp?
Another Inequality by AM GM alone.
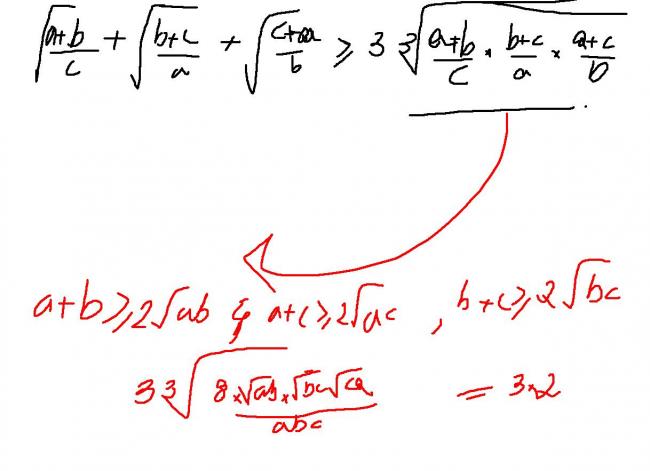
\sqrt{\frac{a+b}{c}}+\sqrt{\frac{b+c}{a}}+\sqrt{\frac{c+a}{b}}\geq 3\sqrt{2}
Hint: AM GM inequality twice.
-
UP 0 DOWN 0 0 10
10 Answers
11i think i got it
but one thing shouldnt the inequality be
>=3 cubeth root of 2
replyy soon
11alrite im posting what i did
applying amgm for the first oneq\geq {2abc+ac(a+c)+bc(b+c)+ab(a+b)}/abc
RHS under power of 1/6
applying amgm to the terms like ac(a+c) we can replace them by 2(ac)3/2
\geq {2+2((ac)^(3/2)/abc+similar terms)}
again applying amgm inequality to the three other terms we get the result
in the second latex equation the term is (ac)3/2/abc
62could you write the whole thing more clearly...
I think you could be quite close or have already solved the problem
1\sum_{cyc}\sqrt{\frac{a+b}{c}}\ge 3(\sqrt{\frac{(a+b)(b+c)(c+a)}{abc}})^{1/3} \\ \text{Now} \\ \frac{a+b}{2} \ge \sqrt{ab} \ [\text{prceeding similarly}] \\ \\ \Rightarrow (a+b)(b+c)(c+a) \ge 8abc \\ \sum_{cyc}\sqrt{\frac{a+b}{c}}\ge 3(\sqrt{(\frac{8abc}{abc}}))^{1/3} \\ \sum_{cyc}\sqrt{\frac{a+b}{c}}\ge 3\sqrt2
