sir where do we take the origin?
Part 1
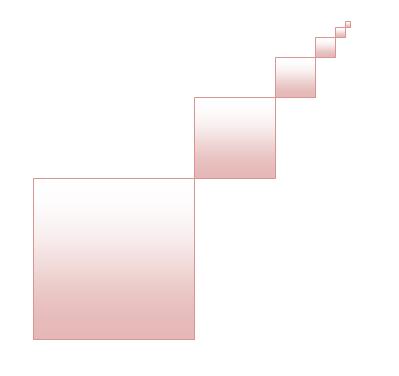
here the radius side of the successive square is half of the original one... (The whole thing goes to infinity)
Side of the Largest square is 1.
Part 2
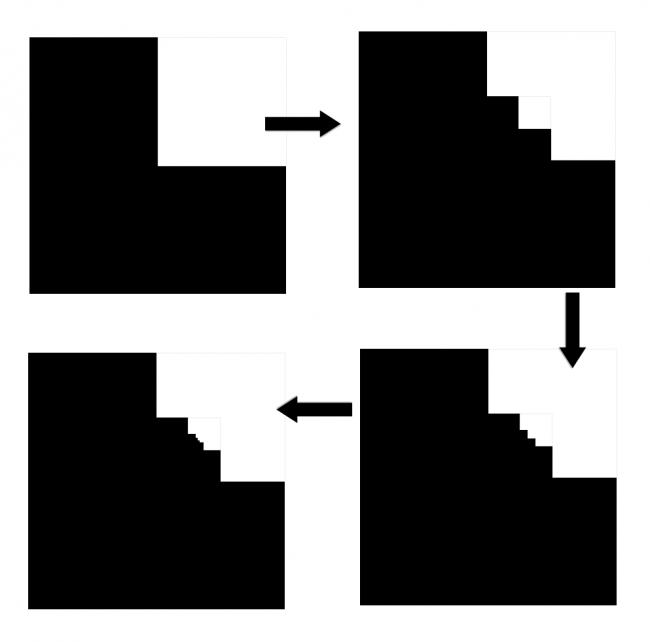
Find the CM of this system...
Here you successively add the same kind of cut squares.. Infinitely many times
First take a square of side one. Remove 1/4th of ti.. then add a square of 1/4th the side of original side and repeat the same thing...
Take the origin as the lower most left point of the bottom square...
-
UP 0 DOWN 0 2 16

16 Answers
Take it whereever you want...
for instance the left bottom most point...
sir:
here the radius of the successive square is half of the original one... ??
R =\frac{ M + \frac{M}{3}(1+R)}{4M/3}
R gives us the x as well as y coordinate of COM
M is mass of the first block
I suppose there is a small error but this was the best method i could think of
Yes I realized as much...
the answer to part I
(57,57)
to part II
(37,37)
____________________________________
for part I
I used
R =\frac{ M.(1/2) + \frac{M}{3}(1+R/2)}{4M/3}
symbols have the same meaning as in #9
similarly for part II
Someone please confirm the answer
PS : I have assumed uniform density
I have not verified the 2nd part... but the 1st one is correct...
can someone post the exact expressoin for the 2nd part?
I just did not want to end this thread here so I left the expression for part II
however here it is
R\, =\, \frac{3M(\frac{5}{12})+\frac{M}{5}(\frac{R}{4}+\frac{1}{2})}{\frac{16M}{5}}
here 3M is the mass of the first block
taken side of largest block as 1
one doubt sir.....is the mass of each square same or is the material same!!!!!