A thread is wrapped over a massless pulley.
The pulley and rope have a coefficient of friction k between them.
If the thread/rope is wrapped by an angle of "theta".. then what is the ratio of the tensions on both sides of the pulley!!
62lol.. yeah the manipulations were correct :)
but how did u get ... or how do u justify:
(T+dT)R -TR = μTRdθ ???
33Yup...
Those friction problems...
A simple principle "pane wale ke pas dene wale se jada nahi hota "
se ban jata hai...
U are talking about 2-3 block one over the other naa?
62hmm.. Priyam.. but i can show you many questions of IIT JEE where using things like
F=μN for friction problems will just kill u(i know u must have seen such questions!)
If u use ≤ instead, you will never get into sup!
btw isnt IIT about "bade log" who have grown a bit from their "bachpan" :P :D
33But its easier to deal with equality than inequality so i solved takin equality and later seeing physical situation introduced inequality..
I always prefer putting -x in place of x for tackling negatives bcause ...
Bachpan me humlog 1,2,3.. sikhe the na ki -1,-2,-3,...:P so more comfortable in +ve and = than -ve and <
1yes i did post exactly this line .. torque due to tensions = frictional torque
but deleted..
thot it will be crap..
i mean as it was a manipulation so i was not sure abt my approach..
33hmmmmm yes...
I myself was not able to understand my approach.. :P
I took so may approximations :P
62not to say that ur method is wrong priyam..
except that at one place u have taken
±dT=μTdθ
but i think the < will come here itself..
bcos F<=μN and not F=μN
62i like sky's approach more.. even though she din say anything after my post..
basically net torque will be I. alpha. But since the pulley is massless, I =0
hence the frictional torque = torque due to tension!!!
hence the result!
33Normal force comes out to be Tdθ and Net force towards ---> is ±dT
where ±dT=μTdθ
T1∫T2dT/T=±μ0∫θdθ
Solving it we get...
T2/T1=e±μθ
Frm it I concluded
e-μθ≤T2/T1≤eμθ
62Because the mistake i made in the question is that i did not mention massless...
Now i edited to correct that :)
62This is not an easy question!!!
Well and most probably not in IIT JEE.
I am taking up this discussion bcos it can be solved by the syllabus in IIT JEE. and bcos of a recent discussion on our chat box.
To be true I dont expect many of you to be able to solve it. Not because it is difficult .. but because the proof is different! (It is used at times in physics.. but rarely)
The hint will follow in my next post... (but i will need a diagram before that .. so will need time..)
1(T+dT)R -TR = μTRdθ
=> T1∫T2 dT/T = 0∫θ μdθ
=> T2/T1 = eμθ
(:P saw the ans... and manipulation... is it corect ?)
62
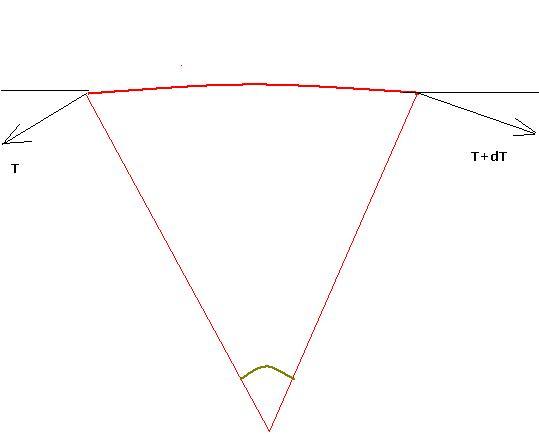
here the lower angle is dθ
The top two angles are dθ/2
now complete this fbd..
and write something!!!!
62lol.. is it.. i dont remember...
i will post this image that i just drew.. for more hints!!
33I think once I asked it U gave the same hint and i solved... :)
Should i post the soln..
I think it asked it before that hanging chain problem (which i had not solved after the hint :P )
33I think once i asked it but it got deleted..
I think the answer was :
e-μθ<T1/T2<eμθ
62
Well now take this .. take tension at the thread on both sides
T and T+dT
And draw the FBD for a small angular element!
I know it is not very simple.. but just give it a shot!