39How is this thermal physics??
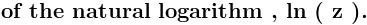
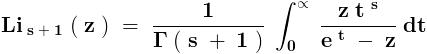
prove
\int_{0}^{\infty}{\frac{x^3}{e^x-1}\mathrm{dx}}=\frac{\pi^4}{15}
-
UP 0 DOWN 0 2 15
15 Answers
1see here
now integrating over all the wavelengths
u encounter that integral
1Plz refer the gamma function on wiki ..for the integrals of the type ∫e-xxn
Limit ranging from 0 to ∞... it will become easy if u also refer
------ R.D Sharma the big book..it has this topic in definite integrals..it is actually easy..if u know how to do the basic qns.
1i said lambda this function is not easy :)..amazing job ricky!!
1well it can be done in a simpler way
.I=\int_{0}^{\infty}{\frac{x^3}{e^x-1}}\mathrm{dx} \\ I=\int_{0}^{\infty}{x^3}\sum_{n=1}^{\infty}{e^{-nx}}\mathrm{dx} \\ I=\sum_{n=1}^{\infty}\int_{0}^{\infty}{x^3}{e^{-nx}}\mathrm{dx} \\ \texttt{NOW we know} \int_{0}^{\infty}{e^{-nx}}\mathrm{dx}=\frac{1}{n}\\ \texttt{differentate thrice w.r.t n}\\ \\ \int_{0}^{\infty}{x^3}{e^{-nx}}\mathrm{dx}=\frac{6}{n^4}\\ \texttt{plug it in I}\\ I=\sum_{n=1}^{\infty}{\frac{6}{n^4}} \zeta (4)=\frac{\pi^4}{90}\\\texttt{this can be proved by tracking down the coefficient of}x^6\texttt{in the expansion of} \\sinx*\sin \i x \\ I=\frac{\pi^4}{15}
courtesy : lectures of physics by feynman [1]
1and the idea of calculating \zeta(4) was stolen directly by basel problem
