Q1. single
With usual notation in triangle ABC, if cos^2\frac{B}{2}+cos^2\frac{C}{2}+cosA = \frac{3}{2}
then sinC + sinB = xsinA.. x =
(a) 5 (b) 3 (c) 4 (d) 2
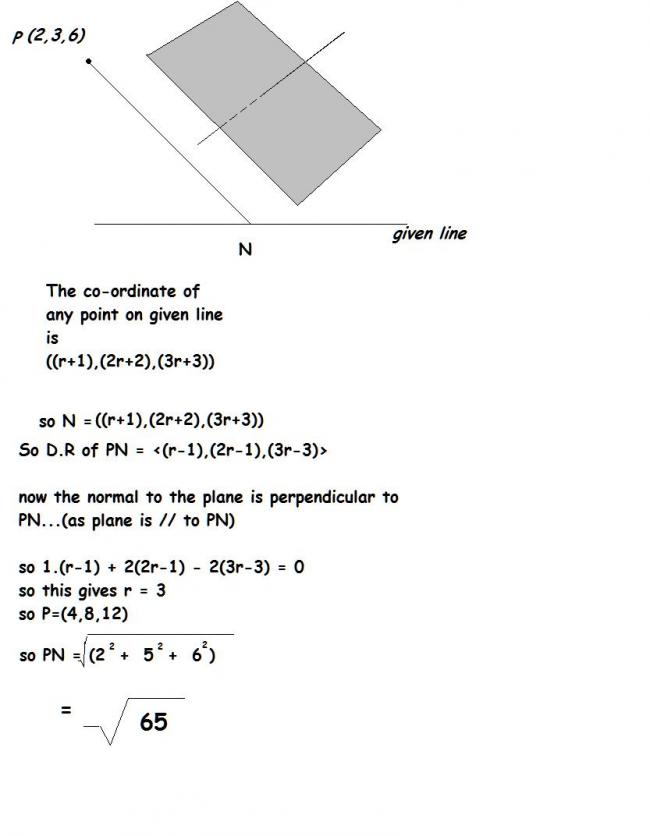
Q2. The distance of the point (2,3,6) from the line \frac{x-1}{1}=\frac{y-2}{2}=\frac{z-3}{3} measured parallel to the plane x+2y-2z+10=0 is
Q3. multi:
Consider that xsinx is positive
xsinxdy+ysinxdx+xcosxydx-x^2dx=0, and y(x=0) = 0, then the value of y at x=pi/2 cannot exceed
(a) 1 (b) 2 (c) 4 (d) 3
Q4. Consider hyperbola S:xy+7x+5y=0. If an ellipse touches the asymptotes of the curve S=0, then the eccentricity of locus of centre of the ellipse is
21Q2)
a). Determine the equation of a plane T passing through P(2,3,6) and parallel to the given plane x+2y-2z+10=0
b) Calculate the coordinates of the point of intersection of the given line and the plane T...
let that point be B
c) the req distance measured parallel to the plane will be BP
11
did you modify the qsn or it came like this only..
in our paper to it was a mcq in ppr 1.
[1]
29Ans3..
xsinxdy+ysinxdx+xcosxydx=x^2dx
now on integrating both sides u will get
xysinx = \frac{x^{3}}{3} + c
Now using the hints given we get c=0
so the equation reduces to
y = \frac{x^{2}}{3sinx}
