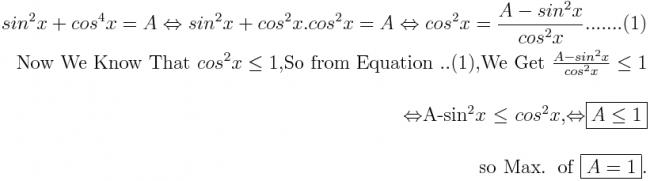EAMCET QUE...

BITSAT Forum › Trignometry questions › EAMCET QUE...

SIN²X + COS⁴X = A

FIND MAXIMUM VALUE OF A....

#Mathematics #Trignometry

UP 0 DOWN 0 0 5
Post on FacebookPost anonymously?

5 Answers

swordfish ·2010-11-07 05:15:52

max value occurs at x = 90/0 degrees
max A = 1

UP 0 DOWN 0
Post on FacebookPost anonymously?

Euclid ·2010-11-07 05:27:58

sin²x + cos⁴x = sin²x + (1-sin²x)² = 1 - 3sin²xcos²x = 1 - 34sin²2x

=> sin²x + cos⁴x = 1 - 34sin²2x

so maxm value = 1

UP 0 DOWN 0
Post on FacebookPost anonymously?

bindaas ·2010-11-07 05:35:01

replace sin²x=1-cos²x
now consider the function
f(x)=x⁴-x²+1
it is an even function
so max value is when cos(x)=1or cos(x)=0 ...(from graph)
=1
the graph of the situation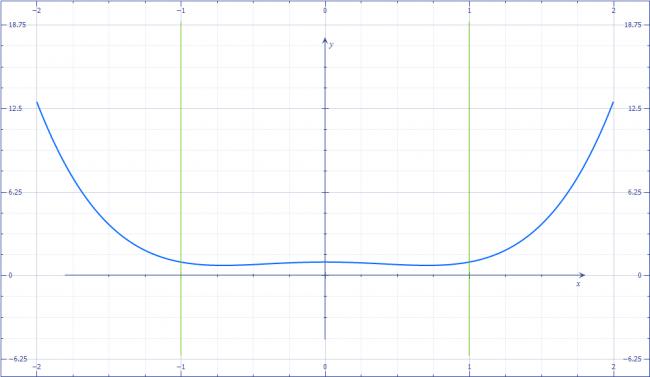

UP 0 DOWN 0
Post on FacebookPost anonymously?

1708

man111 singh ·2010-11-07 10:21:32

UP 0 DOWN 0
Post on FacebookPost anonymously?

Joydoot ghatak ·2010-11-07 19:47:09

see heres another easy method..

sin²x = sin²x.
cos⁴x â‰¤ cos²x.

adding,

sin²x + cos⁴x â‰¤ sin²x + cos²x.
â‰¤ 1.

therefore max value =1.
isnt it a simple method???

UP 0 DOWN 0
Post on FacebookPost anonymously?

Your Answer

Basic Integral Calculus Operators Symbols Matrix Cases

√ √ || {} [] () → ~ ^ x x x → → ln log lg lim lim cos sin tan cot arcsin arccos arctan

∫ ∫ ∫ ∫ ∫ ∬ ∬ ∬ ∬ ∬ ∭ ∭ ∭ ∭ ∭ ⨌ ⨌ ⨌ ⨌ ⨌

∑ ∑ ∑ ∑ ∑ ∮ ∮ ∮ ∮ ∮ ∏ ∏ ∏ ∏ ∏ ∐ ∐ ∐ ∐ ∐

+ - × ÷ ± ∓ = ∪ ∩ ≠ ∼ ≈ ∅ ∀ ∃ ∈ ∋ ∝ ≤ ≥ ≃ ≅ ≡ < > ≪ ≫ ⊂ ∉ ⊃ ⊆ ⊇ ⇒ ⇐ ⇔ ⇒ ⇐ ⇔ ⇑ ⇓ ⇕ → ← ↔ → ← ↔ ↑ ↓ ↕ ↖ ↗ ↘ ↙ ↪ ↩ ⇀ ↼ ⇁ ↽

° ∂ ∞ α β γ δ θ λ μ ν ξ π ρ σ φ ω ε ƒ κ τ υ Γ Δ Λ Σ Π Ω

Close [X]

EAMCET QUE...

5 Answers

Your Answer

Add Image

Similar Questions