now check post
#12
use cauchy schwarz to get it directly
cauchy inequality is
(Σai2)(Σbi2)≥Σaibi2,
but wat sir had chosen ai and bi very cleverly
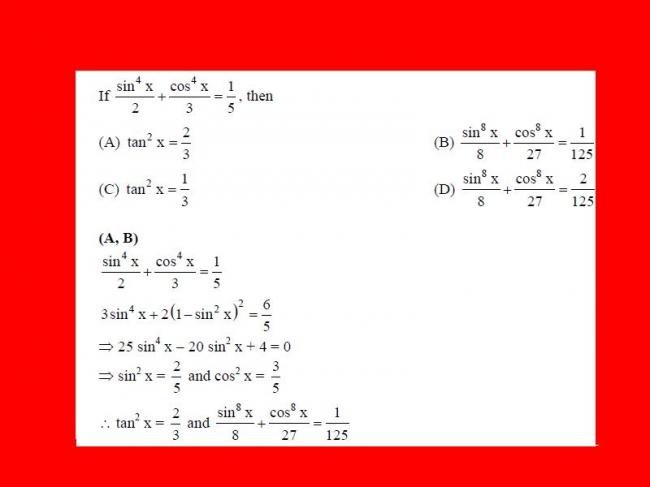
IF
(sin4x)/2+(cos4x)/3=1/5
then
(a)tan2x=2/3
(b)sin8x/8+cos8x/27=1/125
(c)tan2x=1/3
(d)sin8x/8+cos8x/27=2/125???
It's mulit ans question...
-
UP 1 DOWN 0 0 17

17 Answers
cauchy schwarz
could ny one tell about this one please?????????
sorry really sorry i apologise...it is cos/3 nt 2..now please check
hey kalyan the question that u gave is wrong
i also wasted my time yesterday just to get sinof something >1 !
so kindly edit ur question and please check it atleast once before u post
cheers!
@prince i had done the same way......wat makes u say the question is wrong?
the shortest way to do this is by Cauchy Schwarz Inequality (Titu's lemma)
By this inequality we have
\frac{\sin^4 x}{2} + \frac{\cos^4 x}{3} \ge \frac{(\sin^2 x + \cos^2 x)^2}{3+2} = \frac{1}{5} with equality occuring only when
\frac{\sin^2 x}{4} = \frac{\cos^2 x}{9} \Rightarrow \tan^2 x = \frac{4}{9}
Done!!
sin 4x2+cos 4x3= 1/5
3sin 4x + 2(1-sin2x)2 = 6/5
25sin 4x - 20 sin2x + 4 =0
which gives,
sin 2x = 2/5, cos 2x = 3/5
from this we get options (A) and (B) correct.