1) Find √1+2008√1+2009√1+2010√1+2011*2013
2) Find the +ve integers (x,y) which satisfies x2+y2+2xy-2008x-2008y-2009=0
3) an+1 = an + an-1 for n≥2 .
a2 = -1 and a10 = 29. Find a1.
4) Find sum of the digits of the number 100020-20
712.
(x+y)2-2008(x+y)-2009 = 0
=>Say x+y = p (say)
p2-2008p-2009 = 0
Factorizing,
(p-1)(p-2009) = 0
Since p≥0. hence p ≠-1.
Other Solution is x+y = 2009 . From this see how can you fetch the answer.
1.
Proceed like this 2011*2013 = (2012-1)*(2012+1) = 20122-1
Now it's easily done.
713.
Proceed like this
a10 = a9 + a8
=> a10 = 2a8 + a7 .(Think how it came)
Similarly..... go until you get
a10 = 34a2+21a1
You know a10 and a2, Put the values and you get a1 = 3 . (Ans)
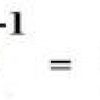1Thanks a lot man
If x+y=2009 and x,y are +ve integers there are 2008 pairs of (x,y) rite?
1708$\boldsymbol{Ans(1):}$\Rightarrow$ $\sqrt{1+2008.\sqrt{1+2009.\sqrt{1+2010.\sqrt{\underbrace{1+2011\times2013}}_{1^{1st}}}}}$\\\\ $\sqrt{1+2011\times2013}=\sqrt{1+(2012-1).(2012+1)}=\sqrt{1+2012^2-1}=\boxed{2012}$\\\\ Now Converted expression is $\sqrt{1+2008.\sqrt{1+2009.\sqrt{\underbrace{1+2010\times2012}}_{2^{nd}}}}$\\\\ $\sqrt{1+2010\times2012}=\sqrt{1+(2011-1).(2011+1)}=\sqrt{1+2011^2-1}=\boxed{2011}$\\\\ further Converted expression is $\sqrt{1+2008.\sqrt{\underbrace{1+2009\times2011}}_{3^{rd}}}$\\\\ $\sqrt{1+2009\times2011}=\sqrt{1+(2010-1).(2010+1)}=\sqrt{1+2010^2-1}=\boxed{2010}$\\\\ Again Converted expression is....\\\\ $\sqrt{1+2008\times2010}=\sqrt{1+(2009-1).(2009+1)}=\sqrt{1+2009^2-1} = \boxed{\boxed{2009}}.$
1708$\boldsymbol{Ans(2):}$\Rightarrow$ $x^2+y^2+2xy-2008x-2008y-2009=0$\\\\ $\underbrace{x^2+y^2+2xy-1}-\underbrace{2008x-2008y-2008}=0$\\\\ $\underbrace{(x+y)^2-1^2}-2008(x+y+1)=0\Leftrightarrow (x+y+1).(x+y-1)-2008(x+y+1)=0$\\\\ $(x+y+1).(x+y-2009)=0$\\\\ Means $x+y+1=0$ and $x+y-2009=0$\\\\ So There are two Paraller line. \underline{\underline{So No solution}}.
1708$\boldsymbol{Ans:(4):}\Rightarrow (1000)^{20}-20=10^{60}-20$\\\\ $Now $10^{60}=1\underbrace{000......00}_{60-digit}$\\\\ So $10^{60}-20 = \underbrace{9999.....9}_{57-digit}80$\\\\ So Sum of Digit is = 9\times57+8\times1+0\times 1= 521$\\\\ $So Sum of Digit in $\boxed{\boxed{(1000)^{20}-20 = 521}}$
1708Yes Shubhodip u rsaying right. There are 2008- pair.
and I have also made amistake in (4).
Ans: = 530.
1708$\boldsymbol{Ans:(2)}\Rightarrow$ $$x^2+y^2+2xy-2008x-2008y-2009=0$\\\\ $\underbrace{(x+y)^2-1^2}-2008\underbrace{(x+y+1)}=0$\\\\ $(x+y+1).(x+y-1)-2008.(x+y+1)=0$\\\\ $(x+y+1).(x+y-2009)=0$\\\\ Means either $(x+y+1)=0$ and $(x+y-2009)=0$\\\\ Here $(x+y+1)\neq0$ bcz $x,y\epsilon Z^+$\\\\ So $x+y-2009\Leftrightarrow x+y=2009$\\\\ So $x=1$ and $y=2008$ Similarly $x=2$ and $x=2007$\\\\......................... $x=2008$ and $y=1$\\\\ So total ordered $\underline{\underline{2008}}$ ordered pair.
