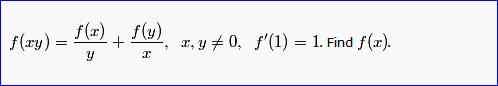Finding f(x)

\hspace{-16}$So $g(1)=0+C=0$(bcz $f(1)=0$)\\\\ So $g(x)=\ln \mid x \mid$\\\\ and $g(x)=xf(x)=\ln \mid x \mid$\\\\ So $f(x)=\frac{\ln \mid x \mid}{x}$ and $x\neq 0$

Yes. That's Correct.
Alternatively (Not very different) , We can directly solve for f(x) by partial derivatives method.

However, Substitution makes the problem look neat.

Nice man111.