STRAIGHT LINES ARE DRAWN by joining m points on a straight line to n points on another line. then excluding the given points , find the points of intersection of the lines drawn
1answer in book is 2xmC2xnC2
now see my method and tel me where i m wrong
total no. of straight lines obtained = mn
now from two lines we get one point. so total no. of points will be mnC2
AND ALSO WE HAVE TO EXCLUDE GIVE (M+N) POINTS.
THEREFORE ANSWER is mnC2-(m+n)
622mC2xnC2
why.. chosing any 2 points on the upper line and any 2 on the lower line will give 2 unique intersection point!
One inside the band of parallel linesl.. another outside..
Let me show the figure corresponding to this one :)
62There is a small requirement.. the points should not be such that a rectangle is formed.. then there will be only 1 point of intersection.
and in that case the answer will be 1 for each 4 points.. otherwise it will be 2
hence the better question could have been to find the maximum no of points of intersection.
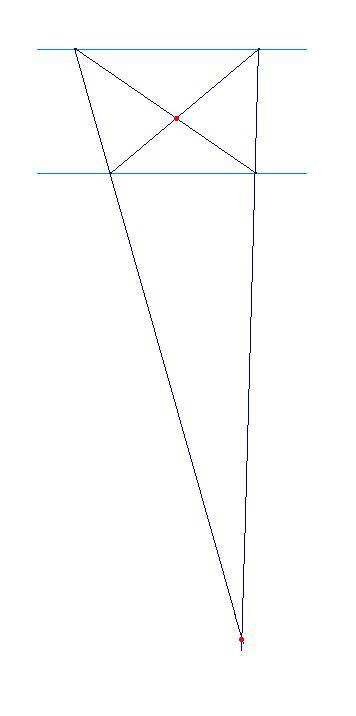
1thanks bro, i got it. can you tel where i had made a mistake
62aman the mistake in your solution is that you have not found out how many times the same point is repeated..
for instance, the first point on the line will be repeated for many cases..
whenever 2 lines are chosen that pass thru the first point.
I hope you got your mistake.
