Suppose we need to solve the equation
x^{x^{x^{x^{.^{.^{.^{.}}}}}}}=2
The usual trick is to realize that the exponent of the bottom x is same the entire LHS, and so we get
x^2=2\qquad \Rightarrow \ x=\sqrt{2}
That is to say that
\sqrt{2}^{\sqrt{2}^{\sqrt{2}^{\sqrt{2}^{.^{.^{.^{.}}}}}}}=2
On the other hand, suppose we had the equation
x^{x^{x^{x^{.^{.^{.^{.}}}}}}}=4
Applying the same trick, we get
x^4=4\qquad\Rightarrow\ x=\sqrt[4]{4}=\sqrt{2}
That is to say that \sqrt{2}^{\sqrt{2}^{\sqrt{2}^{\sqrt{2}^{.^{.^{.^{.}}}}}}} is ALSO equal to 4!?
Question: What is \sqrt{2}^{\sqrt{2}^{\sqrt{2}^{\sqrt{2}^{.^{.^{.^{.}}}}}}} equal to?
1wel im not sure i thought..........xxxx was only equal to unknowns like y.........that is...........
xxxx=y.......then we can subsitute and get it...............but im not sure whether u can use the same for known numbers
1i din't get the question....
but from whatever i cud guess, here's what i think..(dont blame me if its outrageously silly![4])
Let\,\sqrt{2}^{\sqrt{2}^{\sqrt{2}^{....}}} be\,x
\Rightarrow \: \left( \sqrt{2}\ \right)^{x} = x
This is true for both x = 2 and x = 4
Tell me if its correct or not...
1oh...just realized...how can a number be two numbers at once???!!![4][3]
66Yeah.. that is the question.
By the way Akand, how will you solve (for x) the equation
x^{x^{x^{x^{.^{.^{.^{.}}}}}}}=2
1yes kaymant sir i understand.....................but thts d only explanation i cud give for d paradox.................
1I have read this somewhere (ditto same example) in a book. Can I post my reply?
1Please see
http://www.mediafire.com/?sharekey=2cc21860111eb8a2312dbd5f2bdc5062fb70d5567a61ea37ce018c8114394287
33Gaurav says:(For easy viewing i am posting it here...)
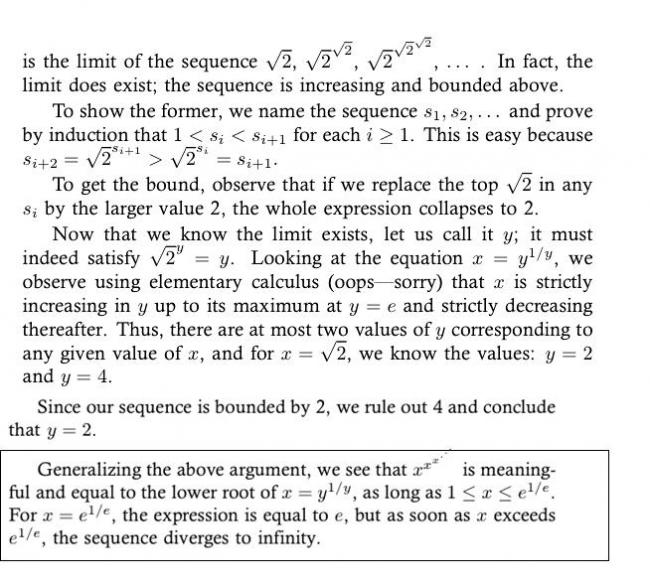
great explanation by (Euler in 1778 ;)...
