Not a QoD.
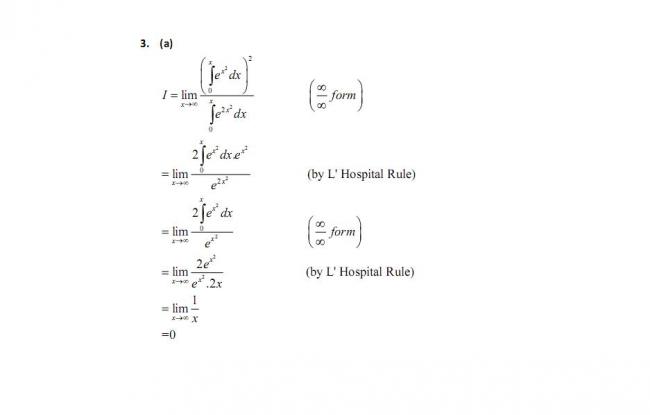
This is of 0/0 form as e∞ is 0. So we apply L'Hospital rule and Newton-Leibnitz theorem together...
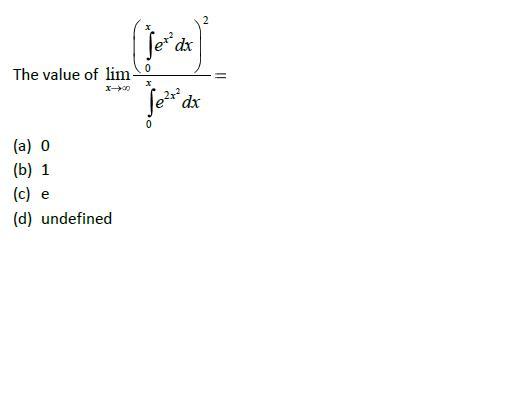
\lim_{x \to \infty} \frac{(\int_{0}^{x}{e^{x^2}dx})^2} {\int_{0}^{x}{e^{2x^2}dx}} = \lim_{x \to \infty} \frac{ 2(\int_{0}^{x}{e^{x^2}dx}) \times (e^{x^2} - 0)} {e^{2x^2} - 0 } = ....
Iske aage koi mahaan insaan aake kar do please.