1first let us take x=y2-1 and y=x-5 and find the points of intersection
putting value of x from 1 in 2
we have y2-y-6=0
(y-3)(y+2)=0
y=3 then x=8
y=-2 then x=3
now let us take x=1-y2
here we get imaginary value of y [4]
22 Answers
1
62\int_{-2}^{3}{(y+5-(y^2-1))dy} - 2\int_{-1}^{1}{1-y^2}dy
={(y^2+5y-y^3/3+y)}|_{-2}^3- 4(y-y^3/3)|_0^1
=9-4+25-27/3-8/3+5 - 4(1-1/3)
= 26
11MERE BHAI ISKA EK CUTTING POINT (8,3) AND DOORA (3,2)HAI
SO HOW COME THE AREA BE 201
I WAS ASKING THIS[7][7][7]
1first let us take x=y2-1 and y=x-5 and find the points of intersection
putting value of x from 1 in 2
we have y2-y-6=0
11YEH Y^2 Y AUR 1 KAHAN SE AAYA
IF U HAVE A NEW METHOD PLEASE POST AND UR ANSWER MAKES NO SENSE
THINK GRAPHICALLY
1\int_{0}^{8}{y^{2}}dy+\int_{0}^{8}{y}dy-\int_{0}^{8}{1}dy =604/3=194.6
62arrey that pink was to show that it is an importantthing to keep in mind :P
removed :P
62
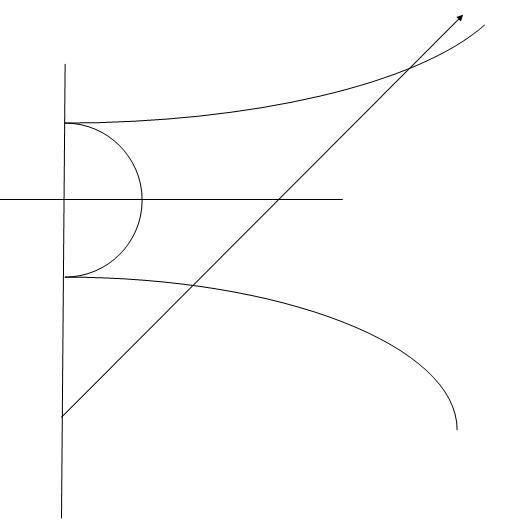
Thi sis one of the most stupid graphs that I have got :D
Or may be I am ;)
what will be the area!
1khud ko hi pink post dete ho..........
buri baat hai ......sheer cheatin' :P
1arey @!#@#%$^%$^# saare kala kari kar er hai
aage badho jawaano :P
11NICCHE WAALA POINT KYA HAI
KALAKARI KARNI BAND KARO
DO SOME WORK
IT WAS DRAWN AGES AGO
11sir itna to ban gaya tha
upper wala point (8,3)
BUT SIR NEGATIVE PE KOI POINT NAHIN MIL RAHA
I MAY BE COMITTING A BLUNDER
PLEASE CORRECT ME [1]
62
without the axis..
I am already hearing that i have made a mistake!