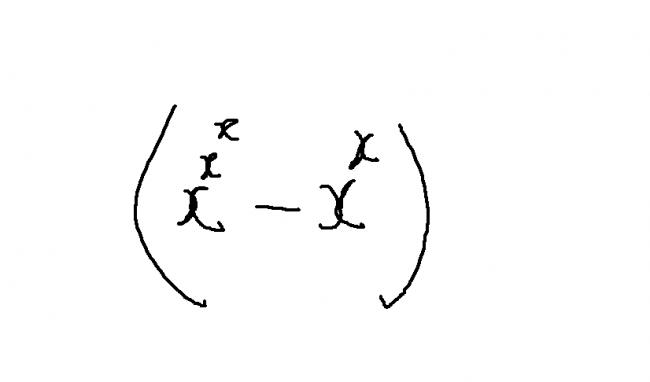21mani....
2 verify ur ans.........
take a value of the order 10^-4 wich is very clos to 0, so u'll get an idea wethr ur ans is rite or not....
13 Answers
21-1
wast da ans bhai jadi batao........
thn i'll post da workinnnnnnnnnn
2121maine bhi..... obviously workin se hi ans aata hai, par this is a meth 2 re-chk ur ans!!!
exam me not useful par here u can do dat............
how will u take log directly as the terms r in subtraction......
I'll post da workin once i get da affirmation regardi my ans's validity.........
24ek part to ho gaya
f(x)=xxx -xx
lim f(x) =lim xxx -limxx
lim xx =L
logL=lim xlogx
logL =lim (logx)/(1/x)
now as x------>0 => logx--->inf and 1/x---->inf =>logL =inf/inf
Aplly L hospital
=> logL={1/x)/(-1/x2)
=>L=1
24limxxx=M
=>logM =lim xxlogx
=>log(log M)=lim (xlogx +log(logx))
=> log(log M)=lim xlogx +lim log(logx)
=> log(log M)=0+lim log(logx)
yahaan tak to theek hai.....
now maybe => M=0 =>lim f(x)=1
21I'll tell my meth........
let t = x^x
y=x^t - t;
logt = xlogx ............(lim x-->0)
= logx/(1/x)......... not defined / ∞
differentiating...........
logt = (1/x)/(-1/x2)
logt = 0
ther4 t=1
y= x^t - t;
applying limits.......
y = 0^1 - 1;
y = 0 - 1
y =-1 [1] [1]
1btw
@tapan ur y can also be written as tx-t.........then limit will be zero
21no chem....
chk properly that wont b the case...........
and neways 0^0 ≠0 as it isnt defined