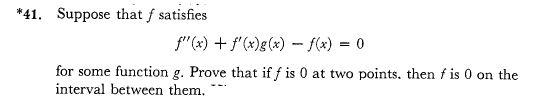dint got the meaing of identically zero
9 Answers
Hint: multiply by e^{\int{g(x)dx}}
also note that e^f is always positive..
let p(x) = f'(x) e∫g(x)dx
p'(x) = f(x) e∫g(x)dx
after that what to do??
btw i had in mind to show f''(x1) >0 and f"(x2)<0 for some x1 ,x2 when f'(x1)= f'(x2) = 0
does that help??
f(x) e∫g(x)dx will be zero at 2 points
so its derivative is zero at some point in between...
Now try...
yeah
taking p(x) = f(x) e∫g(x)dx
p(a)= p(b)=0
from rolles theorem p'(c) = 0 c is somewhere between a and b
so {f'(c) + f(c)g(c) }e∫g(x)dx = 0
giving f'(c) + f(c)g(c) = 0
differentiating once keeping g(c) as a constant
f"(c) + f'(c)g(c) = 0
or f(c)=0 (from given relation)
done?
yup.. it is
now take these two points..
and then prove that there is anotehr point in between where it is zero...
Now what can be proved is that there is an infinitely countable set in the interval where it is zero..
* Going Slightly into higher maths.. We can use the fact that f is continuous and zero on a dense subset of a set (here the interval (a,b)) then it is zero everywhere...
Hence Proved [1]
yeah i knew that f is zero at infinite;y many points...but dint got the term identical...thanks
Let us consider the local maximum of " f " to be occuring at the point " x = a " . Then ,
f ' ( a ) = 0 ................................And f '' ( a ) ≥ 0
Plugging these values in the provided equation ,
f ( a ) = f '' ( a ) ≥ 0 ...............which implies that the minimum value of " f " must be positive .
But that cannot be true since the minimum value ( = 0 ) occurs at the end - points of the interval of which we are concerned .......... ( 1 )
Hence , the minimum value of " f " must be zero as well .
Similarly , by considering the local maxima to occur at " x = b " , we can again prove that the maximum value of " f " in the aforesaid interval must be 0 .......... ( 2 )
Now , ( 1 ) and ( 2 ) cannot simultaneously hold for a non - constant function .
So , " f " must be identically zero in the required region .