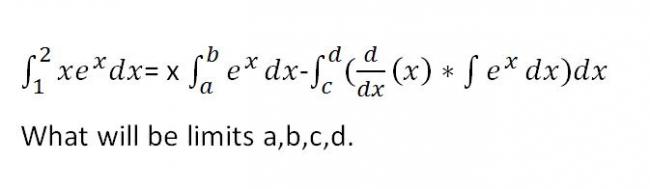Or we have only one choice evaluate the indefinite by parts and take limits at last.
Given, (LL=f(y),UL=f(x))∫et dt = (LL=y,UL=x) ∫1/t dt, for all x,y belonging to (1/e2,∞) where f is continuous and differentiable function and f(1/e)=0.
If g(x)={ ex, x≥k
{ ex2, 0<x<k
Find k such that f(g(x)) is continuous for all x>0.
-
UP 0 DOWN 0 0 20

20 Answers
hmm.. ok
I think there is a mistake in typing or something's still missing cos of partial typing!
I like this one concept..
Good
Thank you abhishek and nishant for the question and solution...
exactly..
no a,b,c,d....
just take their integrals..
and after taht..value 0 and 1!
take teh difference..
yeah i knew this is the doubt u will have..
give me 1 sec.. i will post another image :)
x<0 it is 1/3-x/2
x>1 it is x/2-1/3
in between it is x3/3+1/3-x/2
the trick is that in between (0,1) case c above.. in my post..
u should take integral as sum of integrals from (0,x) and from (x,1)
May be i made some error in the calculation.. but the basic idea is correct... if u do get some different answer tell me.. i will try to correct it :)
Q2
we have to take 3 cases:
a) x<0
b)x>1
c) x ε [0,1]
I hope this is a hint! But i will try to post the graph right away!
I hope u guys try to get the middle paths
pata nahi i'm also getting nothing it is in CPP of FIITJEE sir, which i'm solving
if is a continuous fn for all x except 0 and (LL=0,UL=a)∫f(x)dx=b
If g(x)=(LL=x,UL=a)∫f(t)/t dt, then express (LL=0,UL=a)∫g(x)dx in terms of b
yaar this question does not seem to make too much sense to me!!
Why? bcos we know nothing about f(x)!
Case I
say a=1
f(x)={x} {fractional part of x}
Case II
a=1
f(x) = 1-{x}
B is same for both these cases!
Q.2 Let f(x)= (LL=0,UL=1)∫|t-x|*t dt for all real x. Sketch the graph of f(x) and what is the min value of f(x).
f is a continuous fn for all x except 0 and (LL=0,UL=a)∫f(x)dx=b
If g(x)=(LL=x,UL=a)∫f(t)/t dt, then express (LL=0,UL=a)∫g(x)dx in terms of b
hmm.. i understand what "inspires" u to do differentiate.....
But i strongly think this is not needed in this question...
The first look does not make me feel that there will be different results!
But i am not sure.. i ll check and see :)
what if i differenciate both side of (LL=f(y),UL=f(x))∫et dt = (LL=y,UL=x) ∫1/t dt wrt x then what we will get ...
Hey priyam, have u figured out what f(x) is?
This is simple to do using y=1/e
and the limit of integral from 1/e to x!
so u will get f(x)
The 2nd part is finding k...
If this is the actual blocking point then i will tell u that part as well :)