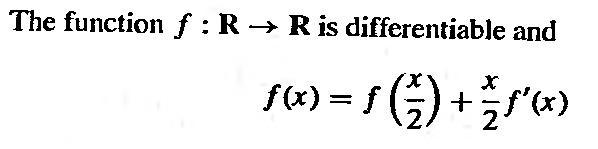function

Hi Kunl
f(x)=f(x2)+x2f'(x)..........(1)
Integrating both sides with respec to x.
∫f(x)dx=∫f(x2)dx +∫xf'(x)dx..........(2)
Let us do this integration
∫f(x2)dx
letx2=t
Differentiating both sides with respect to x
dx=2dt
So∫f(x2)dx=2∫f(t)dt
Now a big thing.
∫f(t)dt=∫f(x)dx
Why?
Because we can change variables as we want.But I am not saying that x/2 =t.But you will see that I do not remove 2.So doing like this is correct.
So∫f(x2)dx=2∫f(x)dx........(3)
From (2) and (3)
∫f(x)dx=2∫f(x)dx+12∫xf'(x)dx
- ∫f(x)dx=12xf(x)-12∫f(x)dx
soxf(x)=-∫f(x)dx
Differentiating both sides with respect to x
xf'(x)+f(x)=-f(x)
xf'(x)=-2f(x)
f'(x)/f(x)=-2/x
Integrating both sides
logf(x)=logk - logx²
So f(x)=k/x²
Great problem.

Two questions for vinay:

(1) Did u verify your solution?

(2) Have you tried f(x) = kx?

Oops!
(1)I did not verify the solution.And after verifying I am getting that the answer that I have got is abject.
(2)I did not try it.But after trying I appreciate it.
But could you see my mistake?