16)A)d=-a
14) B..
13) Period of the function f(x) = sin{sin(∩x)} + e(3x) , where {.} denotes fractional part, is
a)1
b)2
c)3
d)6
14)The min values of the function f(x) = {sin-1(sinx)}2 - sin-1(sinx) is
a)∩/4(Π+ 2)
b)Î /4(Î -2)
c)Î /2(Î +2)
d)Î /2(Î - 2)
16) f(x) = (ax + b)/(cx +d ) , Then for allowable values , fof(x) = x provided that
a) d = -a
b)c = b
c)a=b=1
d)all of these
19) f(x) = x3 + 3x2 + 4x + bsinx + c cosx for all x ε R is a one - one function ,
then the greatest value of b2 + c2 is
a) 1
b)2
c)21/2
d) NONE
20) If 2< x2 < 3 then the no. of +ve roots of {1/x} = {x2} , where {.} denotes the fractional part of x is,
a)0
b)1
c)2
d)3
-
UP 0 DOWN 0 1 9

9 Answers
@Archana : 20 ) ans B
16 ) A, but i was getting all the options true check out once again
14 th Qs is correct ∩ stands for pie (if u dint understand here)
for 16 option A is neccesarily and sufficiently true......and u cant get c to b true
19) f(x) = x3 + 3x2 + 4x + bsinx + c cosx for all x ε R is a one - one function ,
then the greatest value of b2 + c2 is
If it is one-one,
f'(x) = 3x2+6x+4+bcosx-csinx
will have same sign.
Now min. value of 3x2+6x+4 = (4.4.3-36)/4.3 = 1
So, for f'(x) to be +ve always,
min value of bcosx-csinx = -1
this is when b2+c2 = 1
20
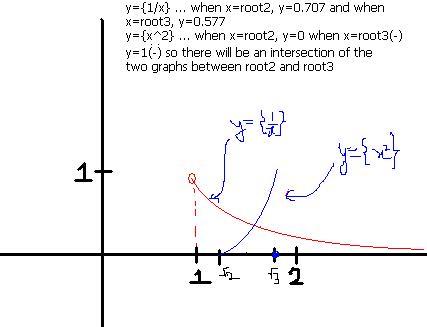
Without graph
y={1/x} is continuous and differentiable for all x ε(√2.√3)
=>dy/dx=-1/x2 which is less than zero for all x>0
=> Monotonically decreasing
y={x2} is alos continuous and differentiable in region (√2.√3)
dy/dx=2x which is greater than zero for all x>0
=> Monotonically increasing
Since these 2 curves are monotonically inc .and dec. respectively
so they must intersect only once in the region