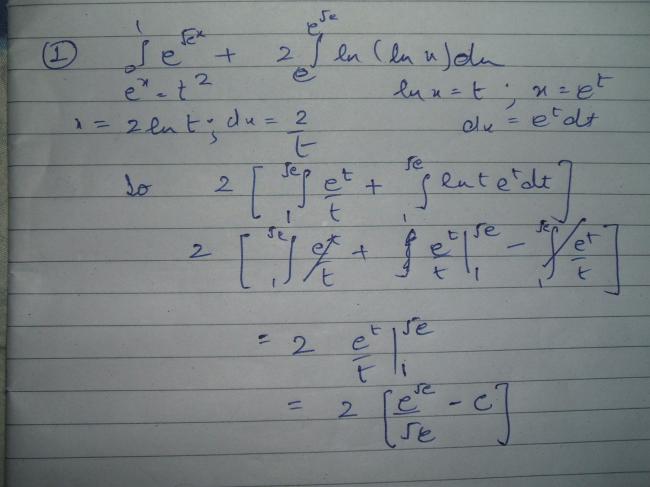this thread is only for integration sums............
AND YA FORGOT TO TELL ALL TARGETIT USERS, I HAVE COPIED ALL THESE SUMS. SO KISIKO GAALI DENA HAIN YA KUCH KEHNA HAIN, PLEASE VISIT
http://targetiit.com/profile711.html AND POST THEM IN CHATBOX. THANKS IN ADVANCE............
1}\int_{0}^{1}e^{\sqrt{e^{x}}}\ dx+2\int_{e}^{e^{\sqrt{e}}}\ln (\ln x)\ dx
2} \int \frac{x^{3}}{(x-1)^{3}(x-2)}\ dx
3}1985 japan women university
\lim_{a\rightarrow + \infty} \frac {\int_0^a \sin ^ 4 x\ dx}{a}
4} \frac{1}{\displaystyle \int _0^{\frac{\pi}{2}} \cos ^{2006}x \cdot \sin 2008 x\ dx}
5} \int_0^{\frac {\pi}{2}} \frac {x^2}{(\cos x + x\sin x)^2}\ dx
6} IF F(X)= x2 + |x| then prove that
\int_{0}^{\pi}f(\cos x)\ dx=2\int_{0}^{\frac{\pi}{2}}f(\sin x)\ dx
7}Evaluate the following definite integral.
\int_{e^{2}}^{e^{3}}\frac {\ln x\cdot\ln (x\ln x)\cdot\ln\{x\ln (x\ln x)\} + \ln x + 1}{\ln x\cdot\ln (x\ln x)}\ dx
8}
Let f a nonnegative ,continuous and periodical function defined on the reals, such that the arithmetic mean of the numbers f(1), f(2),...f(n) tends to zero when n tends to infinity. Prove that f(k)=0 for any natural number k.
9}\int_{0}^{1}\frac {x\ dx}{(x^{2} + x + 1)^{\frac {3}{2}}}
10}\int_{2}^{6}\ln\frac { - 1 + \sqrt {1 + 4x}}{2}\ dx