U mean...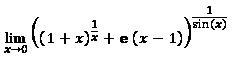
7 Answers
Limit should not exist...
as x→0+ is i think indeterminate (and most prob equal to 0)
but as x→0- it is ∞∞ not indeterminate a very large no i'll say....
hey try L'Hospital..
[(1+x)1/x + e(x-1) ]/sin-1x
This is very simple through that...
u could try taylor series * i havent tried it but i think that will do as well :)
naman were u able to reach any solution after my hint?
Why dont u try L'Hospital.. it will make it very simple.. only the derivation looks a bit tough.. it is not actually :)
hey try L'Hospital..
L= limit x-0 [(1+x)1/x + e(x-1) ]/sin-1x
t=(1+x)1/x
L= limit x-0 [t + e(x-1) ]/sin-1x
L= limit x-0 [t + e(x-1) ]/sin-1x
L= (limit x->0) t/sin-1x + (limit x-0) [e(x-1) ]/sin-1x
(limit x->0) t/sin-1x
dt/dx=t(1/x-1/(x+1) - ln(1+x)/x2 ...... Try getting this!
= t(1/x-1/(x+1)- (x-x2+.. higher order terms)/x2
= t(-1/(x+1) + 1/2 + x(f(x))
now apply LH rule
(limit x->0) t/sin-1x + [e(x-1) ]/sin-1x
= (limx->0) t(-1/(x+1) + 1/2 + x(f(x)) √1-x2 + e
= (limx->0) -e/2 + e
=e/2
typo if nay should by forgiven :)