1a = 1
b = 0.5
is it ?????????
10 Answers
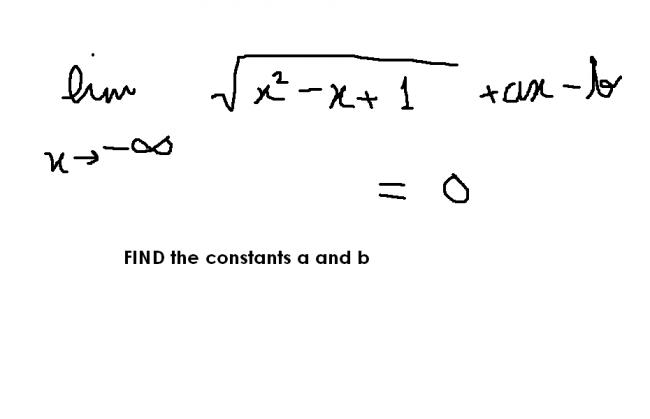
62√x2-x+1 +ax - b
rationalize
{ x2-x+1 -(ax-b)2 }
√x2-x+1 - ax + b
{ x2(1-a2) -x(1-2ab) +1 -(b)2 }
√x2-x+1 - ax + b
Firstly, the numerator should have coeff of x2 as zero...
hence a=±1
{ - (1-2ab)+ (1-b2)/x }
√1/x2-1/x+1 - a + b/x
Now do this using both the cases, a=1 or a=-1
62a=1
{ - (1-2b)+ (1-b2)/x }
√1/x2-1/x+1 - 1 + b/x
{ - (1-2b)+ (1-b2)/x }
√1/x2-1/x+1 - 1 + b/x
for the limit to exist, b=1/2 will give a solutin.. (otherwise it will be of the forum non-zero / zero...
case a=-1
{ - (1+2b)+ (1-b2)/x }
√1/x2-1/x+1 + 1 + b/x
deno is 2 ... (in the limiting case.) .. for numerator to be zero, b=-1/2
Edit*
but when you put back a=1 the original limit is of the form ∞+∞... so that cant be zero...
* end Edit
Hnece
a=1, b=1/2
1sorry...but answer acc to d book is only 1 and .5...........
not the negitive ones.....is dat wrong???
1well,i think nishant bahiyya has solved but didnt checket back by putting the values in original question,
the ans will indeed be a=1 & b = 0.5 only,
the negative ones do not satisfy the org ques....
1just put negative values
lim x->- infty √x2-x+1-x+0.5
u will not get lim = 0