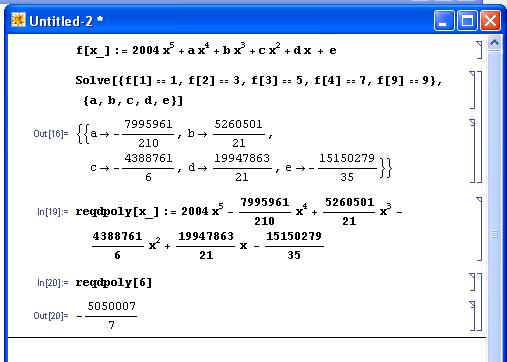
The options given are not correct. The correct answer is f(6)=-\dfrac{5050007}{7}.
To see how its coming, consider the polynomial
Q(x)=f(x)-(2x-1)
Obviously, Q(x) is of degree 5.
Then, we have
Q(1)=f(1)-1=0
Q(2)=f(2)-3=0
Q(3)=f(3)-5=0
Q(4)=f(4)-7=0
That implies that 1, 2, 3, 4 are the roots of Q(x). Since its a polynomial of degree 5, the remaining root must be real as well. Let it be \alpha. Then, taking into account that Q(x) also has the leading coefficient 2004, we can write Q(x) as
Q(x)=2004(x-1)(x-2)(x-3)(x-4)(x-\alpha)
Hence, f(x)=Q(x)+2x-1
i.e. to say that
f(x)=2004(x-1)(x-2)(x-3)(x-4)(x-\alpha)+2x-1
Finally, since f(9)=9, we get
9=2004\cdot 8 \cdot 7\cdot 6 \cdot 5 \cdot (9-\alpha)+17
which gives us
\alpha = \dfrac{3787561}{420840}
Hence, we finally get
\boxed{f(x)=2004(x-1)(x-2)(x-3)(x-4)\left(x- \dfrac{3787561}{420840}\right)+2x-1}
Hence, we get
\boxed{\boxed{f(6)=-\dfrac{5050007}{7}}}