Graph of f ( x ) = √ 1 - x 2 is not a circle . It is the graph of a semicircle above the x - axis .
(1) The no. of solutions of the equation 2^{\left|x \right|}\left(\sqrt{1-x^{2}} \right)=1 is ?
(2)The order of the differential equation y=C_{1}e^{x+c_{2}}+C_{3}e^{x+c_{4}} + C_{5}\sin (x+C_{6})+C_{7}\cos (x+C_{8}) is equal to ?
-
UP 0 DOWN 0 1 4

4 Answers
semicircle is graph of f(x) =√1-x2 ,other curve is of f(x) = 2-|x|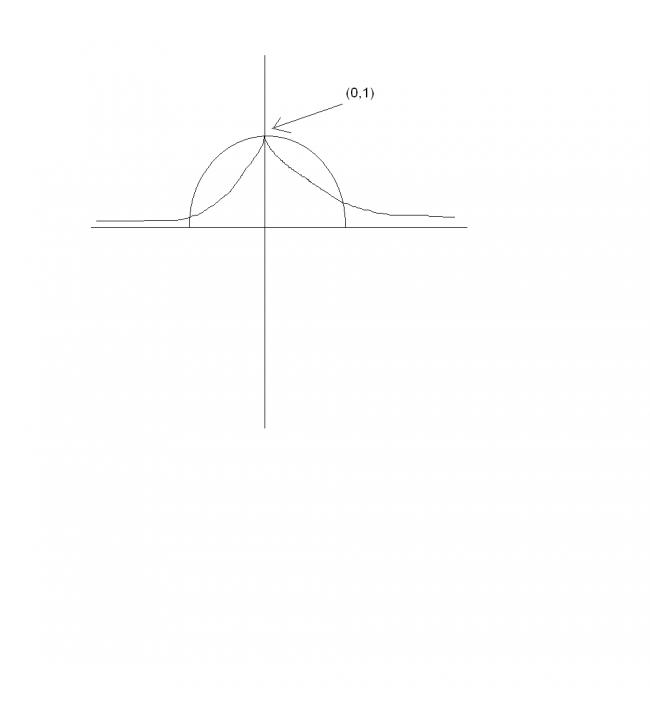
so 3 solutions
1) The graph of f(x)=√1-x2 is a semi circle above the x axis.
2)y=C_{1}e^{x+C_{2}}+C_{3}e^{x+C_{4}}+C_{5}\sin (x+C_{6})+C_{7}\cos (x+C_{8})
\Rightarrowy=C_{1}e^{C_{2}}e^{x}+C_{3}e^{C_{4}}e^{x}+C_{5}\sin (x+C_{6})+C_{7}\cos (x+C_{8})
\Rightarrow y=K_{1}e^{x}+C_{5}\sin (x+C_{6})+C_{7}\cos (x+C_{8})
here K_{1}=(C_{1}e^{C_{2}}+C_{3}e^{C_{4}})
\Rightarrow y=K_{1}e^{x}+C_{5}\left\{\sin x\cos C_{6}+\cos x\sin C_{6} \right\}+ C_{7}\left\{\cos x\cos C_{8} -\sin x\sin C_{8}\right\}
\Rightarrow y=K_{1}e^{x}+K_{2}\sin x+K_{3}\cos x
Hence, there are only three independent constants, so order = 3.