Trigonometric Integral....

We have,

I = \hspace{-16}\mathbf{\int \sin (101\;x).\sin^{99}\;xdx}

I = \int {\sin 100x .\sin^{99}x. \cosx+\cos100x+\sin^{100}x}dx

I = \int \frac{d\;(\sin100x . sin^{100x})}{100 \; dx}\; \; dx

I = \frac{\sin100x . sin^{100x}}{100 } + C

The Idea is not very trivial but I reached there by Hit and trial only. No Logical Solution I could come up till now!

Thanks vivek

i think we can calculate it using Complex no.

By Using Complex No. , Do you mean :

sin (101x) = e^101ix + e^-i101x2i and sin⁹⁹ x = ( e^ix + e^-ix2i )⁹⁹

Plugging that Integrating (Of course not by hand) gives :

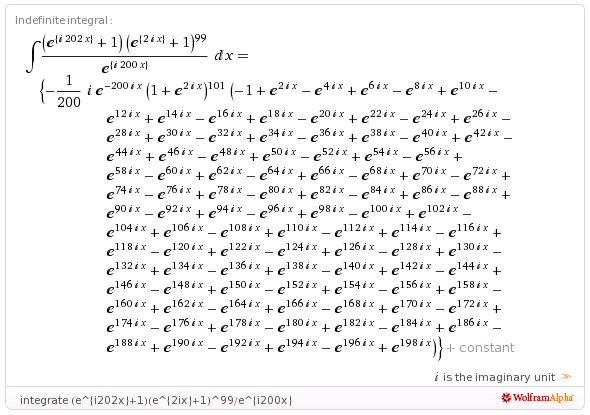
However, I don't think this is the method that you're telling.

Please let me know.

good trail from vivek

yes vivek you are right (very Complex)