30Shouldnt it be BD2=AD.CD ?
6 Answers
21
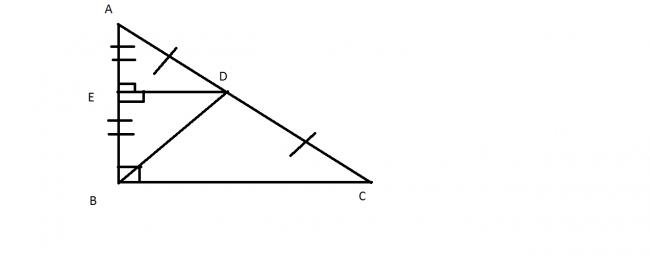
If BD is the median to AC.
AD=DC
Now I have drawn ED||BC.
Then by mid-point theorem, E is the mid-point of AB.
So, AE=EB.
In Triangle ADE and Triangle ABC
AE/AB=AD/AC=1/2
So, Triangle ADE is SIMILAR toTriangle ABC.
Therefore, Angle AED= Angle ABC=90°
In triangle ADE and triangle EDB.
ED is common.
AE=EB (Proved)
Angle AED=Angle DEB=90°(Proved)
So, Triangle ADE is CONGRUENT to Triangle DEB.
So, AD=DB (C.P.C.T.)
So, AD=BD=DC
36@everyone -> Guys i have missed a very important part in my question, BD is the median on AC i.e... AD = CD
36@Arnab -> Why have you used similarity.... i mean you are absolutely right...
But what was the use of similarity...????
Simple :
When you draw DE || BC then LAED = LABC = 900.... (corr. Ls)
and also by Thales' theorm or mid-point theorem...
AE = BE
and so triangles... AED and BED are congruent (by SAS) and so...
BD = AD and so...
AD = BD = CD (simple)