i believe second one is correct clearly first one is wrong
→ → → →
when A * B (A cross product B)= |A||B|sinθn(unit vector)
the direction of the unit vector is taken like this
then my problem is,why the direction of the unit vector is not taken in this direction?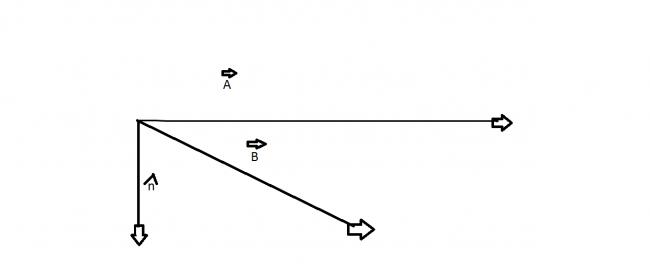
-
UP 0 DOWN 0 0 12

12 Answers
By right hand rule....
Curl your fingers from A towards B (for AXB)...then the direction in which your thumb points is AxB
somewhat like the image below...
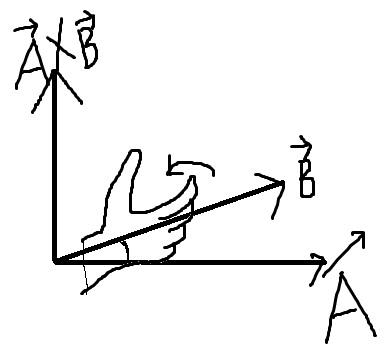
the reason is: because B comes after A....
Jokes apart.. I mean.. AXB points at a particular direction and not in the opposite direction just because human beings who are the "creator" of the cross-product concept have defined the direction to be so...
thus, by definition AXB points in a direction that is given by the method "awesome"-ly explained by Sandipan and there is no other reason..! [1]
think of torque...when u cross product A and B..u get the torque in downward direction...Men have created this operation by seeing the instances..therefore it is defined thus.
to be more precise...when u turn a screw anticlockwise (refer my figure) it comes up.....
and when u turn it clockwise (refer ur 2nd figure) it goes down...
there are two types of "standard" systems being followed left handed and right handed...!so accordingly the definition changes!
AXB ≠BXA
the reason for this is the direction....
when its AXB thn curling of finger starts from A to B..
exactly how sandipan has explained it nd so on..
depends on the figure u r given to deal with....