circles..

IIT JEE 2015 Help › Co-ordinate Geometry questions › circles..

CIrcle C (r=1) touches X axis at A.Centre Q of C lies in Quad I.Tangent from origin touches C at T and P lies on it such that OAP is rt triangle (at A).perimeter =8

Q1 Find PQ
Q2 eqn of circle
Q3 eqn of tangent

here is fig...plz give hints or soln..

#Mathematics #Co-ordinate Geometry

UP 0 DOWN 0 0 2
Post on FacebookPost anonymously?

2 Answers

eragon24 _Retired ·2010-01-26 06:15:03

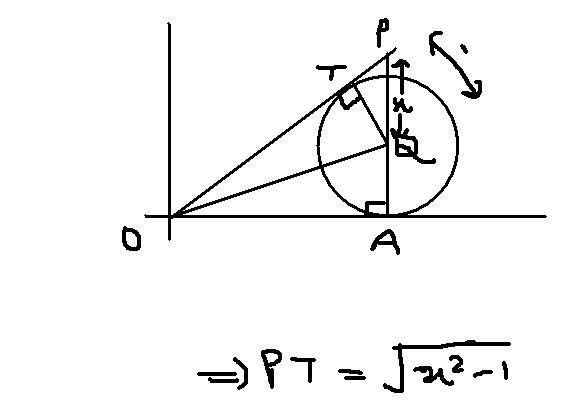
OA=OT=a
PT=b
PQ=x
QA=1
since perimetr=8
so a+b+x+1+a=8..............i

anle QOA=angle QOT=Î²

so tan2Î²=x+1a........ii

tanÎ²=1/a...........................iii

b=âˆš(x²-1).............iv

triangle PTQ is similar to triangle POA

SO a+bx=a1........v

now solve

UP 0 DOWN 0
Post on FacebookPost anonymously?

eureka123 ·2010-01-26 10:07:02

thx...trying now...[1]

UP 0 DOWN 0
Post on FacebookPost anonymously?

Your Answer

Basic Integral Calculus Operators Symbols Matrix Cases

√ √ || {} [] () → ~ ^ x x x → → ln log lg lim lim cos sin tan cot arcsin arccos arctan

∫ ∫ ∫ ∫ ∫ ∬ ∬ ∬ ∬ ∬ ∭ ∭ ∭ ∭ ∭ ⨌ ⨌ ⨌ ⨌ ⨌

∑ ∑ ∑ ∑ ∑ ∮ ∮ ∮ ∮ ∮ ∏ ∏ ∏ ∏ ∏ ∐ ∐ ∐ ∐ ∐

+ - × ÷ ± ∓ = ∪ ∩ ≠ ∼ ≈ ∅ ∀ ∃ ∈ ∋ ∝ ≤ ≥ ≃ ≅ ≡ < > ≪ ≫ ⊂ ∉ ⊃ ⊆ ⊇ ⇒ ⇐ ⇔ ⇒ ⇐ ⇔ ⇑ ⇓ ⇕ → ← ↔ → ← ↔ ↑ ↓ ↕ ↖ ↗ ↘ ↙ ↪ ↩ ⇀ ↼ ⇁ ↽

° ∂ ∞ α β γ δ θ λ μ ν ξ π ρ σ φ ω ε ƒ κ τ υ Γ Δ Λ Σ Π Ω

Close [X]

circles..

2 Answers

Your Answer

Add Image

Similar Questions