34.x=1/2[2x1+(N-1)d]
similar for y both of which are constants.........
hence x^2+y^2 must be equal to a constant........ans must be a circle....is it right???
Swastik Haldar no, the answer is that of a st.line.
1.the radius of the circle whose centre is[-4,0] and which cuts the parabola y2=8x at A and B such that its common chord AB subtends a right angle at the vertex of the parabola is equal to
a.4
b.3
c.√18
d.5
2.a normal is drawn to the parabola y2=4ax at a point P other than the vertex.if it cuts the parabola at point Q, then the least value of OQ, where O is the vertex, is,
a.4√6a
b.2√6a
c.3√√6a
d.NOT
3.tangents PA and PB are drawn to x2=4ay. if mPA and mPB be the slope of these tangents and m2PA+m2PB=4, then locus of 'P' is
a.y2=2x(x-a)
b.y2=x(x-a)
c.y2=2x(2x-a)
d.y2=2x(x-2a)
4.if p,x1,x2... xi ,.. and q,y1,y2,... yi ,.. form two infinite arithmetic sequences with common difference a and b respectively, then find the locus of the point (α,β) where
-
UP 0 DOWN 0 0 2
2 Answers
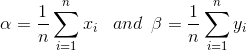
31 must be equal to 5 as no other option looks practical in that sense if u make a diagram
- Swastik Haldar no, the answer is given c.Upvote·0· Reply ·2014-09-09 07:24:15
3