1Thank you Kaymant Sir and Nishant Sir.
The question is clear now. The explanation seems logical to me.
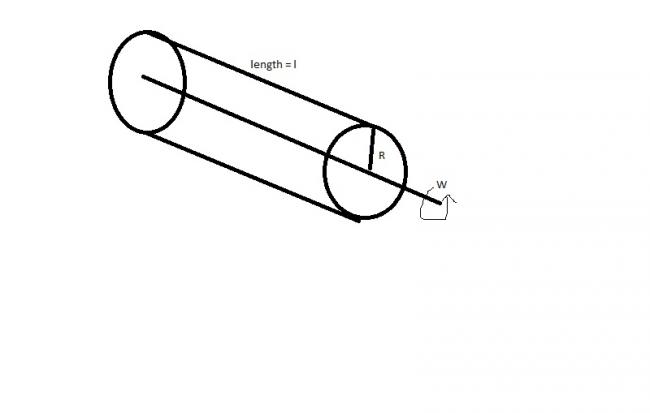
If a cylinder of mass m and length l is rotated about its axis with an angular velocity W, then what is the electric potential difference between the outer surface and the center of the cylinder. The cylinder has no charge initially.
Please consider the cases for both a conductor and a non conductor.
I dont have any idea how to begin in this question. Any help will be appreciated.
-
UP 0 DOWN 0 0 4
4 Answers
1Someone please explain how to begin solving this question. I am stuck.
62in the case of a conductor, the potential difference will be zero.. because there can be no electric field inside a conductor.
What can be done is that assume that there is electric field E(r) at a distance r..
then the force on an electron there will be balanced
Hence E.q = mω2r
Now try to find the find the potential difference as integral of E.dr from 0 to r..
which will be mw2r2/(2q)
66@Nishant Sir,
Your statement "in the case of a conductor, the potential difference will be zero.. because there can be no electric field inside a conductor" is not correct. The electric field is indeed zero inside a conductor but only under equilibrium condition, which is not the case here. In this case there will be a radial field inside the cylinder if it is conducting. Basically, a conductor is modeled as having an endless supply of mobile charges. In case of metallic conductors, these mobile charges are electrons (as can be established via the Hall effect). When the cylinder rotates there would be a redistribution of charges (its the electrons that do the moving) which would generate a field so as to supply the electrons the required centripetal acceleration. That means the field lines must be directed outwards from the axis. The force then on an electron at a distance r is inwards and is given by eE. This should be the same as the centripetal acceleration mω2 r. As such we get the variation of the field as
E(r) = mω2 re.
The potential difference between the axis and circumference is therefore
\Delta V =\int_0^R \dfrac{m\omega^2r}{e}\ \mathrm dr = \dfrac{1}{2}\dfrac{m\omega^2R^2}{e}
On the other hand, for a non-conductor the electrons will be bounded to the lattice and so in this case the required centripetal acceleration comes from the lattice itself resulting in a stress in the lattice. But no potential difference will exists in this case.
1