33It will be
2qR/Ï€(i+j) ...(line joining charge -q and center of arc...)
the dipole moment of a system of charge +q distributed uniformly on an arc of radius r subtending an angle ∩/2at its centre where another charge -q is placed is
(a)2√2qr/∩
(b)√2qr/∩
(c)qr/∩
(d)2qr/∩
soln also needed
-
UP 0 DOWN 0 0 14
14 Answers
33Here also net charge is zero... so considering about -q...
U can do this now... give a try...
11Solution
Charge density on the Arc = Q/ Arc length
Arc LEngth = r*∩/2
C.D =2q/r*∩
Now
P =0∫r(2q/r*∩)*rdx
P = 2q/∩0∫rdx
p = 2qr/∩
11P = ql
where p is dipole moment
q is the charge
l is the length between two charges
3333no!! option (a) is correct...
i am not posting solution... Let her try...
33
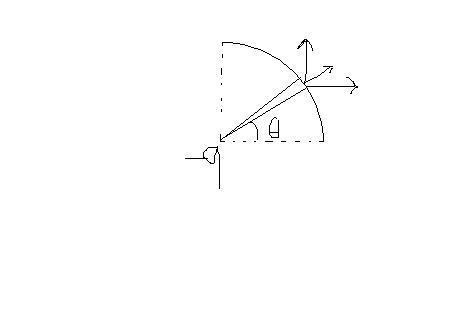
dipole moment
=(2qdθ/π)r
=2qrdθ/π
=2qrdθ/π(cosθi+sinθj) ..(dipole moment is vector..)
integrating from 0 to pi/2
we get...ans
33only thing u missed in ur solution was the point that it is a vector..
and u have to find it about a point...