1yes if u have an arihant book check it out.
18 Answers
1but its given tht the charge is distributed uniformly throughout the sphere,
so the sphere should be non conducting..........
1@ rahul if it is a conducting sphere then the charges will be repelled and will get arranged on the periphery!
1@ Ishan, ques indicates tht
sphere is charged uniformly throughout the volume of the sphere.Then how can electric field at an internal point be 0?
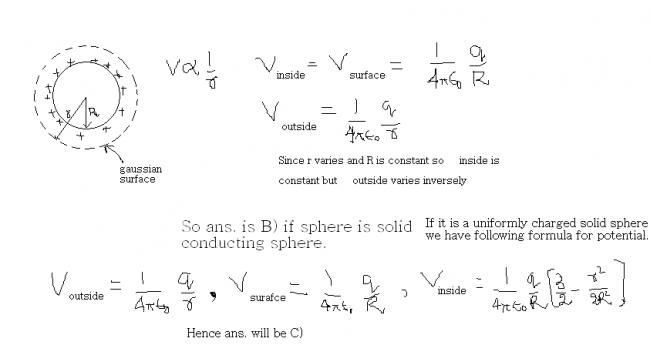
1answer is b (taking the sphereto be conducting)
explaination:
we know that the electric field inside a sphere is zero (shell law which is basically to satisfy the electrostat condition) hence the potential will be constant for r'=0 to r'=r (where r is the radius)
now once we r outside the sphere ,the charge can be considered to be concentrated at the centre of the sphere by spherical symmetry hence it will vary inversely with the square of distance from the centre
graph of a constant function is a straight line parallel to x axis (...i.e when r'<=r)
graph of 1/x2 is a rectangular hyperbola restricted in the first quadrant
join these two and the answer..i.e (B) becomes evident!!
11the ans would had been b if it was a hollow sphere the first line says it is a solid sphere
11take k=1/4(pi)eo
The electric field intensityoutside the sphere is :
Eoutside=k q/r2
dVoutsidedr = -Eoutside
or
\int_{\propto }^{V}{dVoutside} = - \int_{\propto }^{r}{kq/r2} dr
or V = kq/r as V\propto = 0
or V \propto 1/r
At r=R V=kq/R
i.e at the surface of the sphere potential is Vs = kq/R
The electric intensity inside the sphere ,
Einside = k q r/R3
dVinsidedr = - Einside
or \int_{Vs}^{V}{dVinside} = -kqR3\int_{R}^{r}{r dr}
so V - Vs = - kqR3[r22]rR
substituting Vs = kqR(32 - r22R2)
At center r=0 & Vc = (3/2)(kq/R) = 1.5 Vs
i.e. the potential at the center is 1.5 times the potential at the surface
graph will be as my previous post...........
1Eoutside = \frac{1}{4\pi \varepsilon _{0}} \frac{q}{r^{2}} \frac{dV_{outside}}{dr} = - E_{outside} \int_{infinite}^{V}{dV_{outside}} = - \int_{infinite}^{r}{\frac{1}{4\pi \varepsilon _{0}} \frac{q}{r^{2}} }dr V = \frac{1}{4\pi \varepsilon _{0}} \frac{q}{R} at r = R E_{inside} = \frac{1}{4\pi \varepsilon _{0}} .\frac{q}{R^{3}}.r \frac{dV_{inside}}{dr} = - E_{inside} \int_{V_{s}}^{V}{dV_{inside}}= - \frac{1}{4\pi \varepsilon _{0}}\frac{q}{R^{3}}\int_{R}^{r}{r}dr where Vs is potential at surface = \frac{1}{4\pi \varepsilon _{0}} \frac{q}{R}
V - Vs = - \frac{1}{4\pi \varepsilon _{0}} \frac{q}{R^{3}}[\frac{r^{2}}{2}] with integration limits R to r
Substituting Vs, we get V = \frac{1}{4\pi \varepsilon _{0}} \frac{q}{R}\left[\frac{3}{2} - \frac{1}{2}\frac{r^{2}}{R^{2}}] \right
GOT THAT !!!!!
1in the soln i hav , the hint given is that V= k Q(3R2-r2)2R3
HOW HAS THIS BEEN DERIVED???????
