Magnetics

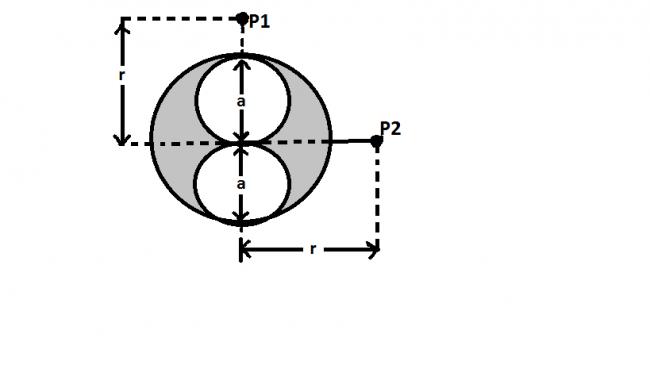
\texttt{Let the current density be J} \\ \\ \mathrm{J=\frac{2I}{\pi a^2}}\\ \\

Now , consider this Cylinder

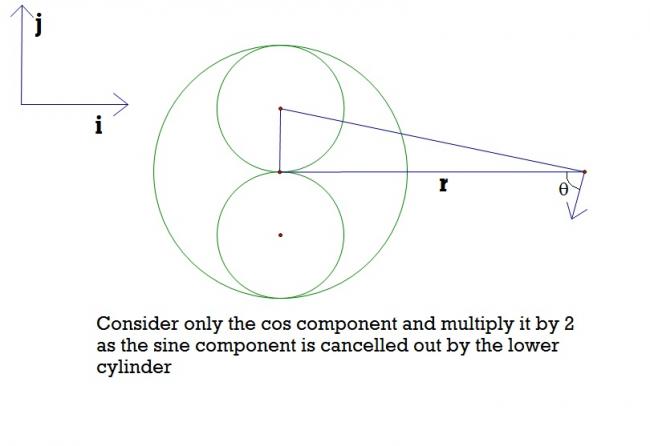
\texttt{Field at Point P1 due to this is } \\ \\ \mathrm{B_1=\frac{\mu_0 2I}{2\pi r}}

The direction is \mathrm{\hat{\theta}}

Now ,consider these two small cylinders one by one
But assume the current is going in the opposite direction
Due to the upper cylinder

\texttt{Field at Point P1 due to this is } \\ \\ \mathrm{B_2=\frac{\mu_0 I}{4\pi (r-\frac{a}{2})}}

Similarly ,consider the lower cylinder

\texttt{Field at Point P1 due to this is } \\ \\ \mathrm{B_3=\frac{\mu_0 2I}{4\pi (r+\frac{a}{2})}}

But B2 and B3 are in \mathrm{-\hat{\theta}}

So,net effet is
B1+B2+B3

B=\frac{\mu_0 I}{\pi}\left(\frac{1}{r}-\frac{1}{4}\left(\frac{1}{r-\frac{a}{2}}+\frac{1}{r+\frac{a}{2}} \right) \right)

Similarly , approach for 2nd one

its simple:
B=(mu*I)/(2*pi*R)
fo
r both the pts