1
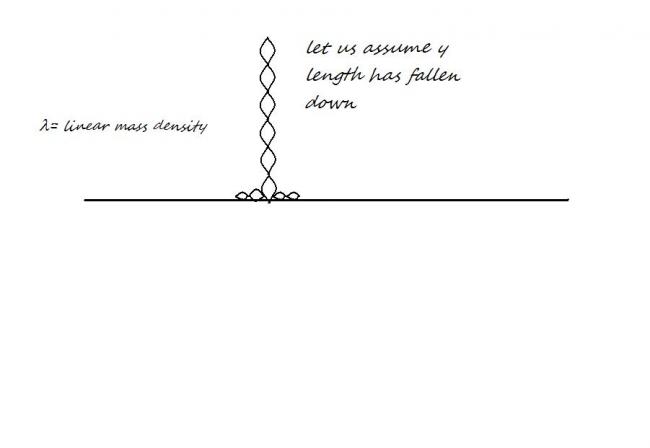\\\texttt {mass of the falling part is clearly }=\lambda(L-y)\\ \\\texttt{the thrust force means here , like the ground must apply some force to stop the momentum of chain } \\\texttt{, u can see the part , just before touching the ground has some velocity} \\\texttt{and then it becomes 0 suddenly , the impulse provided by the ground does it all }\\\ F_{thrust}=\frac{d\lambda(L-y)}{dt}.v_r \\ F_{thrust}=-\lambda\frac{dy}{dt}.v_r \\ \frac{dy}{dt}=v_r \\ F_{thrust}=\lambda v_r^2 \\ v_r^2 =2gy \\\texttt{this is coming from basic kinematics } \\ F_1=\lambda2gy \\\texttt{now the force exerted by the ground on already lying mass on table is }\\ F_2=\lambda gy \\ F_1=2F_2 \\ \\\texttt{hence proved }
u just need to realise the ground 's normal force has to do two jobs
1) balnce the weight thats lying
2) stop the momentum of the the end that just touches it
if u realise the second point , the thrust force comes into the play
and then u can easily solve the problem