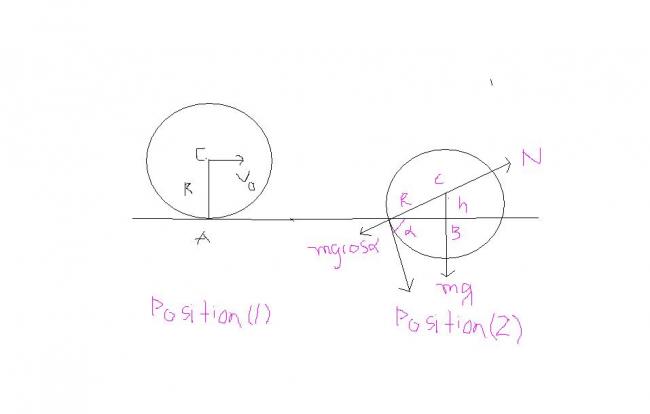
Question., A uniform solid cylinder of radius R=15cm rolls over a horizontal plane passing into an inclined plane forming an angle α=30 deg with the horizontal downwards. Find the max. value of the velocity vo which still permits the cylinder to roll onto the inclined plane section without a jump. The sliding is assumed to be absent. 
19use these facts:
during transition from horizontal to inclined plane:
(i) centre of cylinder i.e. its centre of mass rotates , thus centrifugal force comes nito play
(ii) direction of Normal force on cylinder from the plane rotates
(iii) for rolling without a jump, N≠0...thus for the limiting condition, we should use N=0
19i am too lazy to do that !
find N and its direction,,,equate it with other forces and bla..bla...bla !
19what more do u want ? any problems to proceed further ?? you can show your steps if the answer is not matching !
1look, I wrote the equation when the cylinder went to another plane:
N+ mv2R=mgcosθ.
by this I am getting v=1.14m/s..............if N=0 for limiting case.
BUT, the answer in IRODOV is v=√13gr(7cosθ-4) =1m/s
GOD knows how it came.
Please someone explain how this answer came.
1total M.E at position 1 is
E1=mgR +0.5IAωA=
=mgR +0.5(1.5mR2)(v0/R)2
total M.E at position 2 is E2 =mg(BC)+0.5(1.5mR2)(vC/R)2
BUT BC +Rcosα
now, E1 =E2 .............(1)
also centre of mass moves on circular path about o at position2
mgcosα-N=M(vC/R)2
FOR NOT JUMPING N>0
mgcosα>N ............(2)
THUS WE GET
v0MAX.=√(gR/3)(7COSα-4)
PUTTING VALUE WE GET 1M/S
1Thanks abhishek,
but in writing ur equation of E1 and E2 ,you were not taking kinetic energy of the cylinder. WHY????????????
21@biswajit in finding E1 since we r taking MOI of the cylinder from an axis through point A which is the instantaneous axis of rotation so we can assume cylinder to be rotating abt point A hence having only rotational energy.........if u wud hav taken MOI from the axis of the cylinder passing through the centre of the cylinder then u wud hav to consider KE and rotatinal kinetic enegry separetly.......similarly for E2
total KE of cylinder or a disc or a sphere =(1/2)Ipw2
where Ip is MOI throgh IAOR
