33Find max tension in the string first...
Two bodies of mass m and 4m are attached with string.Body of mass m length l(String) is executing oscillation of amplitude θ while other is at rest.The min coeff of friction between 4m and ground should be.....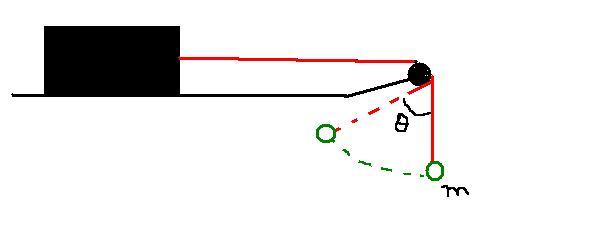
-
UP 0 DOWN 0 0 15
15 Answers
33T=mv2/l+mg.cosα
write v in terms of α and then differentiate it to find α for max T and then that max T will help U further..
:)
33Should i post complete soln... ??
In b/w u r in which class??
24but why are we differentiating ?????Why is T not max at amplitude of oscillation?
And about my class I will correct it very soon........
33bcause T depends on two things v2 and cos(angle) so if u say at amplitude it is max but how v=0 then and cos() is also small..
well a wild guess it should be at mean position bcause there v is also max and cos() is aslo max i.e. 1
so try with mean position..
24ooops sorryy......didnt think about that......ok tryng .........have u reached the answer????
33[3] not solving it just seeing and replying..
actually i am downloading something so in between that am having a peep here
[3]
But if u want i can solve it..
24okkk solve it.....maybe i am making mistake while diff........i am getting absurd answer.
24no u arent sleepy........maybe i am sleepy thats why i am making mistakes.....give ur soln ......
33T is max at lowest point as i explained above:
it should be at mean position bcause there v is also max and cos() is aslo max i.e. 1
so by writing energy conservations mgl(1-cosθ)=mv2/2
we get mv2=2mgl(1-cosθ)
so as T=mv2/l+mg.cosα...............(α=0 as lowest point)
there fore T=mg(3-2cosθ)
and T=μmin4mg
hence the answer..
:)