fjee aits - rotation

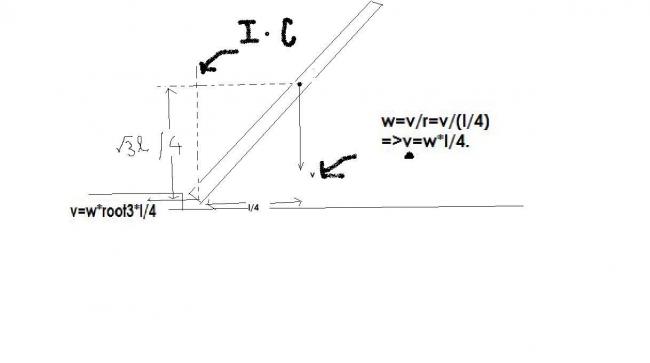
Vcom=ωl/4 ....(because velocity of lowest point is only along plane..) ----> din get [2] [2] [2] [2]

also by instantaeneous center method v=wl/4

hey am sry 4 not understanding :P but for last two i have been constantly finding out wrong ans ... n am fedup wid my rusted mind [2]

but y wl/4 ?

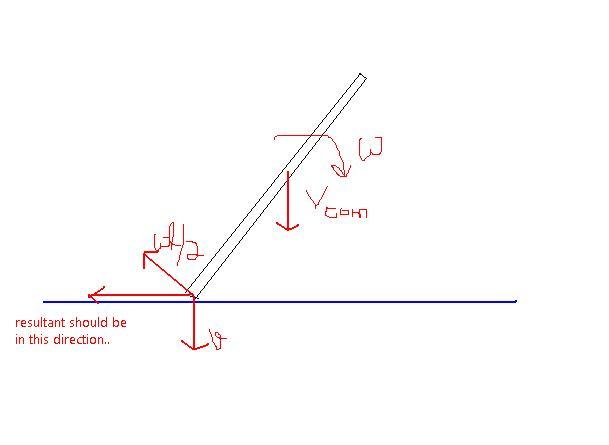
cant we conserve angular momentum about point P???

mvl/2 = I_pw ??

plz correct if wrong...

2.) the angular velocity of the rod just afetr its lowest point collides with P is :

a) g/4

b) g/2

c) √21g/8

d) √21g/32

○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○

V_com=ωl/4 ....(because velocity of lowest point is only along plane..)

mgl/4(2-√3)=1/2mv_com²+1/2ml²/12ω²...

Put v_com and value of l to get answer√24g/7..

[1]

__________

added later to complete the soln..

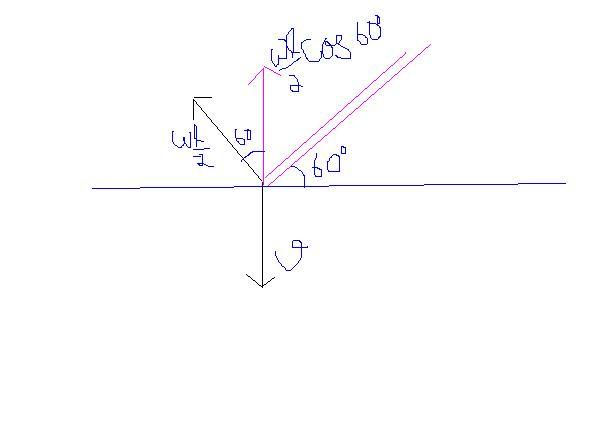
now is it clear...
ωl/2cos60⁰=v
v=wl/4..

○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●○●

mgl(1/2-√3/4)=K.E.

You have a doubt or this is for others..[7]

ans for 2 >>[d]