1
ok, i know the solution. but it shows that pressure at point A and B will be same. because we havn't considered height
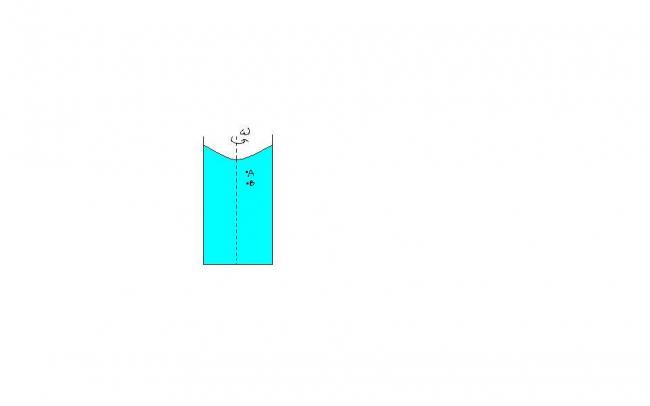
A liquid of density p is in bucket that spins with angular velocity w (omega). show that the pressure at radial distance 'r' from the axis is
P = P° + pw2r2/2
where P° is the atmospheric pressure
-
UP 0 DOWN 0 0 4
4 Answers
62Aman this is an easy problem
Hint: Take the elementary mass on the surface.
Take distance r.
Its acceleration to the center is ......
Draw the FBD.
Equate!
Sorry if this seems to be a stupid hint. But this is all that u have to do :)
162Aman I think you have made a wrong interpretation of the question.
I dont think that is what the question says.
It will be different at A and B. There is no doubt about that! It is only to find the pressure at the depth of the crest. I mean at the lowermost point of the parabolic part .. not the lowest point of the bucket!