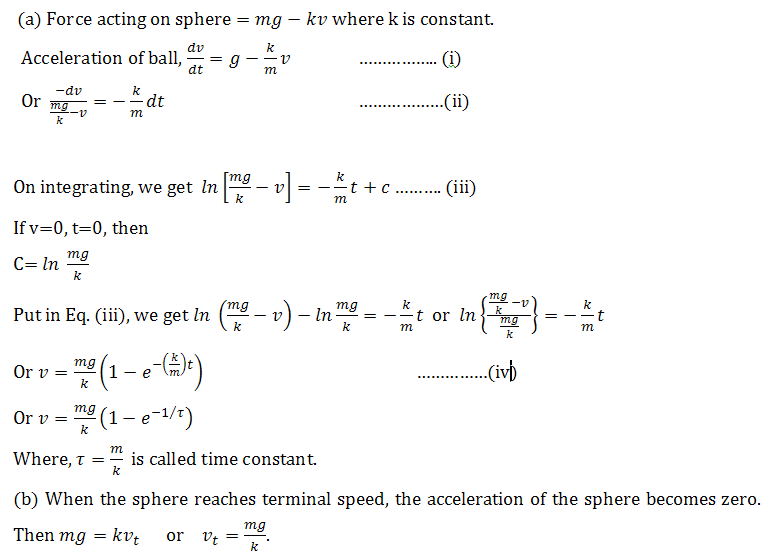
A small sphere of mass m is released from rest in a large vessel filled with oil where it experiences a resistive force proportional to its speed i.e., F=-kv, where k is a constant and v is the velocity.
(a) Find the speed of the ball with which it varies.
(b) After a certain time the sphere reaches a terminal speed, find it.
-
UP 0 DOWN 0 0 3
3 Answers
1133a) v = e-ktm
b) v = mgk(1 - ÏlÏs)
Akash Anand do we really need rho-l and rho-s. Can't we do this sum without using that ?? ThinkkkkUpvote·0· Reply ·2013-06-11 06:31:55- Sourish Ghosh Is the first part correct? Do we have to find v as function of time?
- Akash Anand Yeah you have to ...according to the condition provided
- Sourish Ghosh Any hints?
1133Alright here's what I have done. Where am i going wrong?
When body attains terminal vel (vt), net force is zero.
F = -kv
=> mdvdt = -kv
on solving,
v = e-kt/m
a = -kme-kt/m
mg = Fb + kvt
=> Fb = mg - kvt
At any general point before terminal velocity,
mg + ma = Fb + kv
=> ma = k(v - vt)
on substituting values,
vt = 2e-kt/m
- Akash Anand You are wrong at putting the limits of first integration, you should get a constant and then you gonna get the value of that constant. That will be the expression for velocity.
- Sourish Ghosh But, at t = 0, v = 0
- Akash Anand Just try to put the limits properly once again ..whatever you are saying is correct.
- Sourish Ghosh ok if i put limits then
v/u = e^(t-t0)/k
but u is zero :/
1161Here is the solution
- Sourish Ghosh Is F = -kv, the buoyant force?