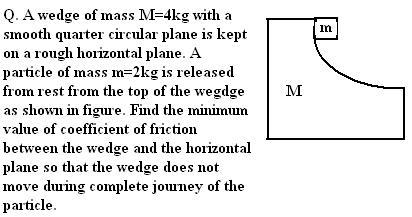
well here are the steps . .
first step find the velocity of m as a function of θ (angle to horizontal) (by conservation of energy)
the normal reaction in their interface will increase compensating mv2/R + mgsinθ
the force on M along horizontal direction will be backwards = Ncosθ
the normal force on ground= Nsinθ+Mg
so we have for the wedge to not slip
Ncosθ<=μ(Nsinθ+Mg)
from here we will get μ as a inequality in θ (as we know N and v in terms of θ)
for the wedge to not slip at each moment μ should be greater than the maximum value of the corresponding left expression
.. thus you will arrive at the answer