1I suppose, the answer shud be,
A-r, B-p,r
Reason, R is the general definition for work, when the force is acting aong line of motion, cos@=1, and would still be valid,
But for p in terms of A, the object may come back to the same position, making the fs curve weird, where in the area under the curve will not give work....
Considering the graph, only where force supports or opposes motion, the graph comes out to be.....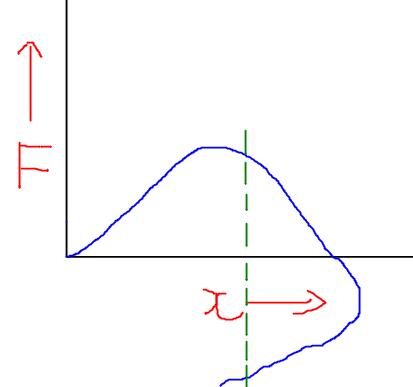
This becomes more complicated when force may act in any direction...
So in my point of view, A-p will not be valid.
please correct me for any errors
[339]
11See Simple explanation
In the first force the direction and Angle of force application is not given
F.S curve ka area is for cos 0. Therefore r will be only ∫F.S Where F and S are vector.
Second one is given that angle of application is 0 Therefore F.S curve and ∫F.S Where F and S are vector. are rite therefore option p,r
1Yeah thats how I did it, but I thought graph would make it clear!!
