11Wow Vivek, tumhara doggie toh bahut cute hai [3]
9 Answers
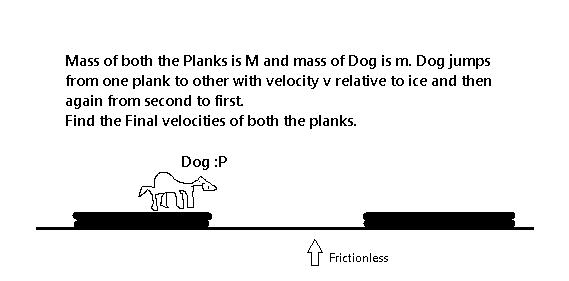
11the second plank will have momentum of (M+m)v
the first plank will have momentum of -Mv
1I am getting final velocity of the plank on right as m2v/M(m+M).
Please verify if it is correct. I shall post the solution only then
I like your dog very much. Which species does it belong to? [4]
71:P I don't have answers, Only question. BTW, I solved it too, but because of lack of answers, I need to confirm.
106Initial jump from left plank
Pi = 0
Pfdog = mv (i)
So, vfplank = -mv/M (i)
Dog reaching second plank
Pi = mv (i)
Pf = (M+m)v'
=> v' = mv/(M+m) i
Dog jumps from second plank
Pi = mv (i)
Pfdog = -mv (i)
vfplank = 2mv/M (i)
Dog reaches first plank
Pisystem = -mv -mv (i) = -2mv (i)
vfsystem = -2mv/(M+m) (i)
Final vel. of first plank (left one ) = 2mv/(M+m) (to left)
Final vel. of second plank = 2mv/M (to right)
hoping ive nt made any calc. errors
1Asish bro...
I think there is one mistake--
Even I got the same till here
"Initial jump from left plank
Pi = 0
Pfdog = mv (i)
So, vfplank = -mv/M (i)
Dog reaching second plank
Pi = mv (i)
Pf = (M+m)v'
=> v' = mv/(M+m) i"
Now,
Dog jumps from second plank
Pi = mv (i)
Pfdog = -mv (i)
vfplank = 2mv/M (i)
Here you are not considering the initial momentum of the plank which is
mv/(M+m) towards right w.r.t ground.
71Here you are not considering the initial momentum of the plank which is
mv/(M+m) towards right w.r.t ground.
But Why.. during the second jump the velocity of the second plank should have increased more na.
@Khyati
Kitni mehnat se banaya tha... Daar raha tha kahi 5 pair na ban jaye! :)
1During the second jump,
Treating dog and plank as our system,
Pi(dog) + Pi(plank) = Pf(dog) + Pf(plank)
m(mv/M+m) + M(mv/M+m) = -mv + Mv1
I think it should be done this way.
106@swordfish , if u analyse the LHS of the eqn obtained .. ull see that it is the same as wat ive written
my logic was that :
before the dog jumped frm second plank , let the P of system be P1.
when the dog was about to jump onto second plank, let the P of system be P2
P2 = mv.
It suffices to see that P1 = P2