1I did it by another method
35 Answers
1Moment of inertia ==
∫r2dm where r is the _|_ distance of mass dm from axis of rotation
does this help
find lower two rods MOI that will be easy
then for above two think _|_ dist not get distracted due to bhaiyya's mistake MOI for both above one s will be equal so you can
multiply by 2....
should I post the MOI of the system...
62yeah so i am calculating it perpendicular to the plane..
or am i sleeping :D
could you give the method philip?
1sorry if i done any mistake bhaiyya but since you ask for it..
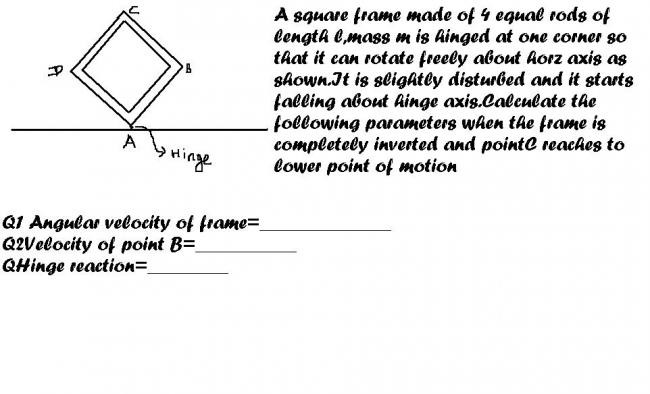
it is to be understood that the lesser mortal who solved this question in his own very messy way assumed that the horizontal line was to be taken as the axis of rotation and while falling the square will come out of the plane of the screen
so MOI of two rods below taken together
ml2/3 (i.e. ml2/6 x 2)
and for the above any one rod..
MOI = ml2/24 + m(9h2/4) where h = l/√2
(in this step a marked difference is to be noted that if the axis be coming out of your screen Nishant bhaiyyas method is completely correct.. .)
so MOI = ml2/24 + 9ml2/8
editing further...
so total MOIsystem = 7ml2/3+ ml2/3 ....
rest can be done ..
it is to be noted that this method might contain many things which are wrong but as always the person is eager to know whether something needs correction [6]
1my method was so bad that no one is even looking at it [2]
and others are deleting their posts.. [2][2]
well i am listening for corrections ....
post them in my chat box if you want to avoid looking at the method above....
62@philip
where did u get ml2/24??
it is ml2/12 na?? even if u take m/4 then u get ml2/48!!
am I sleeping or something is seriously wrong with me :D
1sir jee nahi galat hai pura galat hai upar wale he pehle vale mein galtiya hai..
this time you have messed it up bhaiyya :D
MOI will be ml2/(12sin2θ) :D
1Consider an axis passing thru the centre of the square,for which u'l get moi=4ml2/3
Now consider another axis which is diagonal to the square,then by perpendicular axis theorem,u'l get it to be 2ml2/3
now using parallel axis theorem ,for this axis and the horizontal axis,
moi=8ml2/3.
62oops finally i got my mistake :)
tx guys :)
Now i can sleep well :)
62Wrong solution :)
first calculate teh moment of inertia of the system about A
that will be given by
m{ l2/3 + l2/3 + l2/12 + 5/4l2 + l2/12 + 5/4l2 }
= m{ 20/6 l2 }
= 10/3 ml2
now for first part,
conservation of energy will give
1/2. (10/3 ml2)ω2 = 4mgl√2
ω2 = 12√2/5 g/l
Seems a very strange answer to me..
is it correct eureka?
24sir answer is wrong.......[2]......
one doubt.....why mass =m/4 in MOI ???[12]
62
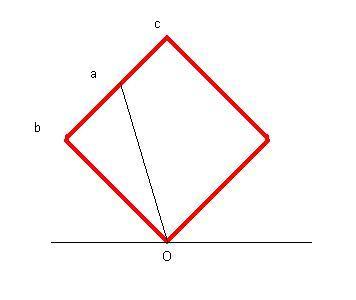
now on this image, for the rod BC
moment of inertia about a is given by ml2/12
moment of inertia about O is given by ml2/12 + m(OA)2
OA2=l2+(l/2)2
Wrong solution :)
24oh yes....but answer is still not matching..........
options are:
a)√(3g/l√2)
b)√(3g/l)
c)√(3g√2/l)
d)√6g/l
62i dont seem to have an answer..
i must be making some stupid mistake :(
21part 1 : conserve energy u'll get it.... { make sure u calc correct PE by taking ht of ind COM }
11i have a doubt have you taken moi for horizontal axis
i think they are for the axis ppr to the plane
1yes i believe MOI to be wrong even though it might not be for the reason subhash gave...
ok lets see..........(will post answer after 5 mins)