1i am gettind velocity of upper end is 4m\sec!
angularvelocity of rod = 1
and angularmomentum as = 25m/2.
it ti right!
i have prob in third answer!!
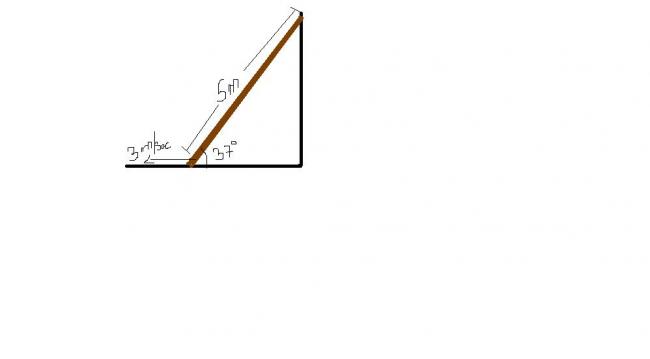
there is a rod of length 5 m.
it is leaning or inlcined to a wall with one end on ground and upper end on the wall!
it makes angle 37* with tthe ground!
below end is given velocity 3m in horizontal direction!
find.
velocity of upper end .?
angular velocity of rod?
angular momentum of the rod?
-
UP 0 DOWN 0 0 13
13 Answers
1
62let the lengths be l, b
l2+h2=c
2l.dl/dt+2h.dh/dt=0
db/dt = -(l/h) dl/dt
=- cot(37). dl/dt
dl/dt is 3.. now you can solve this part 1...
62sorry din see ur reply...
Angular momentum is found from a fixed point....
So you have to find I about a fixed point and ω wrt that fixed point...
The fixed point here can be taken as the point which is perpendicular to the direction of the motion of both the ends of the rod...
which is like found on completion of the rectangle with one vertex at the vertex of the wall and the other two at the ends of the rod....
See if this hint helps?
1did'n get you!
is this right!
i think ....
angular momentum is = rxp
now
r(centre of mass) = - 2i + 3/2j.
mv= m( -3i -4j)
so
L=m ( rxv)
=25/2k x m
= 25m/2..
62The isssue is that angular momentum has to be found about a point....
You have not mentioned the point!
1about the point where the ground and wall meet!
takint it as (0,0).
33A very traditional problem with many solns..
First way >
as Nishant bhaiya did... l2+h2=c
then find velocity...
33Third method >
Work done by internal force is zero... U can get it from here also...
All same one with only different appearance though
1another method is there...
write the coordinates of the points at which the vel has to found..
write the relation of it with the point whose vel is given.
then differentiate, u get the vel relation.
33Another one find axis of rotation and then ω and then velocity of req point..
[3]
5 same methods..