1is it A???
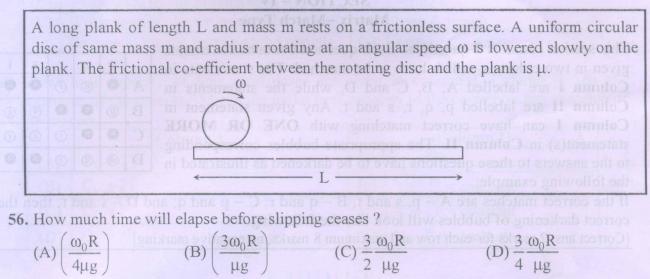
PS: i dont hav d ans ...so pls post full method ....its not a lengthy one :P
-
UP 0 DOWN 0 0 10
10 Answers
1nice sum dude
....
the fore acting on the sphere is umg in the forward direction since the roling is in the opp direction......
and the force on the plank is umg in th e backwards direction
now the equation part at the time of rolling
for the sphere
vsphere=ugt (in the right direction)
w=w-umgrt/I (obviously i can be cacluated(
for the plank
vplankis ugt (in the left direction)
now for no slipping wr-vsphere=vplank
solve and get the asnwer and abt my method i am sure that its right
23in the frame fixed to d plank ...
linear accn a of disc = 2\mu g
fr=I\alpha
\mu mgr=I\alpha
\alpha =\frac{2\mu g}{r}
w=w_{o}-\alpha t
v=at
v=rw
\frac{v}{r}=w_{o}-\alpha t
2\mu gt=w_{o}r-2\mu gt
t=\frac{w_{o}r}{4\mu g}
BUTTT
i posted dis sum here bcz i wanted to actually ask that in my above methd ..is the value of \alpha correct?? will its value change in the frame fixed to d ground ??
23but xyz varun tinkles solution gives d ans ....he has worked in an inertial frame ..
23i myself got confused about the slipping conditions in te ground frame ...hence using the planks frame to use v=rw directly makes it simple!!
1if it is given that a body is in translation equilibrium and suppose torque about a an axis comes zero then the torque about any point
(provided body is in translation equilibrium)
is zero or not ?
[source NCERT XI class]
1but it is zero because when it is given that body is in translation equilibrium then torque does not depend on the point about which torque is calculated